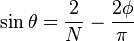Phased Array - Mathematical Perspective and Formulas

Mathematical Perspective and Formulas

A phased array is an example of N-slit diffraction. It may also be viewed as the coherent addition of N line sources. Since each individual antenna acts as a slit, emitting radio waves, their diffraction pattern can be calculated by adding the phase shift φ to the fringing term.

We will begin from the N-slit diffraction pattern derived on the diffraction formalism page.

\psi ={{\psi }_0}\left(\frac{\sin \left(\frac{{\pi a}}{\lambda }\sin\theta \right)}{\frac{{\pi a}}{\lambda }\sin\theta}\right)\left(\frac{\sin \left(\frac{N}{2}{kd}\sin\theta\right)}{\sin \left(\frac{{kd}}{2}\sin\theta \right)}\right)

Now, adding a φ term to the fringe effect in the second term yields:

\psi ={{\psi }_0}\left(\frac{\sin \left(\frac{{\pi a}}{\lambda }\sin \theta\right)}{\frac{{\pi a}}{\lambda }\sin\theta}\right)\left(\frac{\sin \left(\frac{N}{2}\big(\frac{{2\pi d}}{\lambda }\sin\theta + \phi \big)\right)}{\sin \left(\frac{{\pi d}}{\lambda }\sin\theta +\phi \right)}\right)

Taking the square of the wave function gives us the intensity of the wave.

I = I_0{{\left(\frac{\sin \left(\frac{\pi a}{\lambda }\sin\theta\right)}{\frac{{\pi a}}{\lambda } \sin \theta }\right)}^2}{{\left(\frac{\sin \left(\frac{N}{2}(\frac{2\pi d}{\lambda} \sin\theta+\phi )\right)}{\sin \left(\frac{{\pi d}}{\lambda } \sin\theta+\phi \right)}\right)}^2}
I =I_0{{\left(\frac{\sin \left(\frac{{\pi a}}{\lambda } \sin\theta\right)}{\frac{{\pi a}}{\lambda } \sin\theta}\right)}^2}{{\left(\frac{\sin \left(\frac{\pi }{\lambda } N d \sin\theta+\frac{N}{2} \phi \right)}{\sin \left(\frac{{\pi d}}{\lambda } \sin\theta+\phi \right)}\right)}^2}

Now space the emitters a distance apart. This distance is chosen for simplicity of calculation but can be adjusted as any scalar fraction of the wavelength.

I =I_0{{\left(\frac{\sin \left(\frac{\pi a}{\lambda } \sin\theta \right)}{\frac{\pi a}{\lambda } \sin\theta }\right)}^2}{{\left(\frac{\sin \left(\frac{\pi }{4} N \sin\theta+\frac{N}{2} \phi \right)}{\sin \left(\frac{\pi }{4} \sin\theta+ \phi \right)}\right)}^2}

As sine achieves its maximum at, we set the numerator of the second term = 1.

\frac{\pi }{4} N \sin\theta+\frac{N}{2} \phi = \frac{\pi }{2}
\sin\theta=\left(\frac{\pi }{2} - \frac{N}{2} \phi \right)\frac{4}{N \pi }
\sin\theta=\frac{2}{N}-\frac{2\phi }{\pi }

Thus as N gets large, the term will be dominated by the term. As sine can oscillate between −1 and 1, we can see that setting will send the maximum energy on an angle given by

Additionally, we can see that if we wish to adjust the angle at which the maximum energy is emitted, we need only to adjust the phase shift φ between successive antennas. Indeed the phase shift corresponds to the negative angle of maximum signal.

A similar calculation will show that the denominator is minimized by the same factor.

Read more about this topic:  Phased Array

Famous quotes containing the words mathematical, perspective and/or formulas:

    “All science requires mathematics. The knowledge of mathematical things is almost innate in us.... This is the easiest of sciences, a fact which is obvious in that no one’s brain rejects it; for laymen and people who are utterly illiterate know how to count and reckon.”
    —Roger Bacon (c. 1214–c. 1294)

    “Egoism is the law of perspective as it applies to feelings, according to which what is closest to us appears to be large and weighty, while size and weight decrease with our distance from things.”
    —Friedrich Nietzsche (1844–1900)

    “That’s the great danger of sectarian opinions, they always accept the formulas of past events as useful for the measurement of future events and they never are, if you have high standards of accuracy.”
    —John Dos Passos (1896–1970)