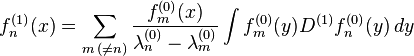Perturbation Theory - First-order Non-singular Perturbation Theory

First-order Non-singular Perturbation Theory

This section develops, in simplified terms, the general theory for the perturbative solution to a differential equation to the first order. To keep the exposition simple, a crucial assumption is made: that the solutions to the unperturbed system are not degenerate, so that the perturbation series can be inverted. There are ways of dealing with the degenerate (or singular) case; these require extra care.

Suppose one wants to solve a differential equation of the form

where D is some specific differential operator, and is an eigenvalue. Many problems involving ordinary or partial differential equations can be cast in this form. It is presumed that the differential operator can be written in the form

where is presumed to be small, and that furthermore, the complete set of solutions for are known. That is, one has a set of solutions, labelled by some arbitrary index n, such that

.

Furthermore, one assumes that the set of solutions form an orthonormal set:

with the Kronecker delta function.

To zeroth order, one expects that the solutions are then somehow "close" to one of the unperturbed solutions . That is,

and

.

where denotes the relative size, in big-O notation, of the perturbation. To solve this problem, one assumes that the solution can be written as a linear combination of the :

with all of the constants except for n, where . Substituting this last expansion into the differential equation, taking the inner product of the result with, and making use of orthogonality, one obtains

This can be trivially rewritten as a simple linear algebra problem of finding the eigenvalue of a matrix, where

where the matrix elements are given by

Rather than solving this full matrix equation, one notes that, of all the in the linear equation, only one, namely, is not small. Thus, to the first order in, the linear equation may be solved trivially as

since all of the other terms in the linear equation are of order . The above gives the solution of the eigenvalue to first order in perturbation theory.

The function to first order is obtained through similar reasoning. Substituting

so that

gives an equation for . It may be solved integrating with the partition of unity

to give

which gives the exact solution to the perturbed differential equation to the first order in the perturbation .

Several important observations can be made about the form of this solution. First, the sum over functions with differences of eigenvalues in the denominator resembles the resolvent in Fredholm theory. This is no accident; the resolvent acts essentially as a kind of Green's function or propagator, passing the perturbation along. Higher-order perturbations resemble this form, with an additional sum over a resolvent appearing at each order.

The form of this solution is sufficient to illustrate the idea behind the small-divisor problem. If, for whatever reason, two eigenvalues are close so that difference become small, the corresponding term in the sum will become disproportionately large. In particular, if this happens in higher-order terms, the high-order perturbation may become as large or larger in magnitude than the first-order perturbation. Such a situation calls into question the validity of doing a perturbation to begin with. This can be understood to be a fairly catastrophic situation; it is frequently encountered in chaotic dynamical systems, and requires the development of techniques other than perturbation theory to solve the problem.

Curiously, the situation is not at all bad if two or more eigenvalues are exactly equal. This case is referred to as singular or degenerate perturbation theory. The degeneracy of eigenvalues indicates that the unperturbed system has some sort of symmetry, and that the generators of the symmetry commute with the unperturbed differential operator. Typically, the perturbing term does not possess the symmetry; one says the perturbation lifts or breaks the degeneracy. In this case, the perturbation can still be performed; however, one must be careful to work in a basis for the unperturbed states so that these map one-to-one to the perturbed states, rather than being a mixture.