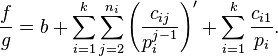Partial Fraction - Application To Symbolic Integration

Application To Symbolic Integration

For the purpose of symbolic integration, the preceding result may be refined into

Let ƒ and g be nonzero polynomials over a field K. Write g as a product of powers of pairwise coprime polynomials which have no multiple root in an algebraically closed field:

There are (unique) polynomials b and c ij with deg c ij < deg p i such that
\frac{f}{g}=b+\sum_{i=1}^k\sum_{j=2}^{n_i}\left(\frac{c_{ij}}{p_i^{j-1}}\right)' + \sum_{i=1}^k \frac{c_{i1}}{p_i}.
where denotes the derivative of

This reduces the computation of the antiderivative of a rational function to the integration of the last sum, with is called the logarithmic part, because its antiderivative is a linear combination of logarithms.

Read more about this topic:  Partial Fraction

Famous quotes containing the words application to, application, symbolic and/or integration:

    “It would be disingenuous, however, not to point out that some things are considered as morally certain, that is, as having sufficient certainty for application to ordinary life, even though they may be uncertain in relation to the absolute power of God.”
    —René Descartes (1596–1650)

    “It is known that Whistler when asked how long it took him to paint one of his “nocturnes” answered: “All of my life.” With the same rigor he could have said that all of the centuries that preceded the moment when he painted were necessary. From that correct application of the law of causality it follows that the slightest event presupposes the inconceivable universe and, conversely, that the universe needs even the slightest of events.”
    —Jorge Luis Borges (1899–1986)

    “Play permits the child to resolve in symbolic form unsolved problems of the past and to cope directly or symbolically with present concerns. It is also his most significant tool for preparing himself for the future and its tasks.”
    —Bruno Bettelheim (20th century)

    “Look back, to slavery, to suffrage, to integration and one thing is clear. Fashions in bigotry come and go. The right thing lasts.”
    —Anna Quindlen (b. 1952)