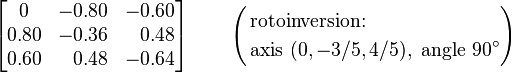Orthogonal Matrix - Examples

Examples

Below are a few examples of small orthogonal matrices and possible interpretations.

  • \begin{bmatrix} 1 & 0 \\ 0 & 1 \\ \end{bmatrix} \qquad (\text{identity transformation})

An instance of a 2×2 rotation matrix:

  • R(16.26^\circ) = \begin{bmatrix} \cos \theta & -\sin \theta \\ \sin \theta & \cos \theta \\ \end{bmatrix} = \begin{bmatrix} 0.96 & -0.28 \\ 0.28 & \;\;\,0.96 \\ \end{bmatrix} \qquad (\text{rotation by }16.26^\circ )
  • \begin{bmatrix} 1 & 0 \\ 0 & -1 \\ \end{bmatrix} \qquad (\text{reflection across }x\text{-axis})
  • \begin{bmatrix} 0 & -0.80 & -0.60 \\ 0.80 & -0.36 & \;\;\,0.48 \\ 0.60 & \;\;\,0.48 & -0.64 \end{bmatrix} \qquad \left( \begin{align}&\text{rotoinversion:} \\&\text{axis }(0,-3/5,4/5),\text{ angle }90^{\circ}\end{align}\right)
  • \begin{bmatrix} 0 & 0 & 0 & 1 \\ 0 & 0 & 1 & 0 \\ 1 & 0 & 0 & 0 \\ 0 & 1 & 0 & 0 \end{bmatrix} \qquad (\text{permutation of coordinate axes})

Read more about this topic:  Orthogonal Matrix

Famous quotes containing the word examples:

    “There are many examples of women that have excelled in learning, and even in war, but this is no reason we should bring ‘em all up to Latin and Greek or else military discipline, instead of needle-work and housewifry.”
    —Bernard Mandeville (1670–1733)

    “It is hardly to be believed how spiritual reflections when mixed with a little physics can hold people’s attention and give them a livelier idea of God than do the often ill-applied examples of his wrath.”
    —G.C. (Georg Christoph)

    “In the examples that I here bring in of what I have [read], heard, done or said, I have refrained from daring to alter even the smallest and most indifferent circumstances. My conscience falsifies not an iota; for my knowledge I cannot answer.”
    —Michel de Montaigne (1533–1592)