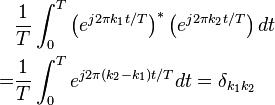Orthogonal Frequency-division Multiplexing - Mathematical Description

Mathematical Description

If sub-carriers are used, and each sub-carrier is modulated using alternative symbols, the OFDM symbol alphabet consists of combined symbols.

The low-pass equivalent OFDM signal is expressed as:

\ \nu(t)=\sum_{k=0}^{N-1}X_k e^{j2\pi kt/T}, \quad 0\le t<T,

where are the data symbols, is the number of sub-carriers, and is the OFDM symbol time. The sub-carrier spacing of makes them orthogonal over each symbol period; this property is expressed as:

\begin{align} &\frac{1}{T}\int_0^{T}\left(e^{j2\pi k_1t/T}\right)^* \left(e^{j2\pi k_2t/T}\right)dt \\ = &\frac{1}{T}\int_0^{T}e^{j2\pi (k_2-k_1)t/T}dt = \delta_{k_1k_2} \end{align}

where denotes the complex conjugate operator and is the Kronecker delta.

To avoid intersymbol interference in multipath fading channels, a guard interval of length is inserted prior to the OFDM block. During this interval, a cyclic prefix is transmitted such that the signal in the interval equals the signal in the interval . The OFDM signal with cyclic prefix is thus:

The low-pass signal above can be either real or complex-valued. Real-valued low-pass equivalent signals are typically transmitted at baseband—wireline applications such as DSL use this approach. For wireless applications, the low-pass signal is typically complex-valued; in which case, the transmitted signal is up-converted to a carrier frequency . In general, the transmitted signal can be represented as:

\begin{align} s(t) & = \Re\left\{\nu(t) e^{j2\pi f_c t}\right\} \\ & = \sum_{k=0}^{N-1}|X_k|\cos\left(2\pi t + \arg\right) \end{align}

Read more about this topic:  Orthogonal Frequency-division Multiplexing

Famous quotes containing the words mathematical and/or description:

    “What is history? Its beginning is that of the centuries of systematic work devoted to the solution of the enigma of death, so that death itself may eventually be overcome. That is why people write symphonies, and why they discover mathematical infinity and electromagnetic waves.”
    —Boris Pasternak (1890–1960)

    “An intentional object is given by a word or a phrase which gives a description under which.”
    —Gertrude Elizabeth Margaret Anscombe (b. 1919)