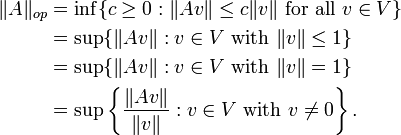Operator Norm - Equivalent Definitions

Equivalent Definitions

One can show that the following definitions are all equivalent:

$\begin{align} \|A\|_{op} &= \inf\{c \ge 0 : \|Av\| \le c\|v\| \mbox{ for all } v\in V\} \\ &= \sup\{\|Av\| : v\in V \mbox{ with }\|v\| \le 1\} \\ &= \sup\{\|Av\| : v\in V \mbox{ with }\|v\| = 1\} \\ &= \sup\left\{\frac{\|Av\|}{\|v\|} : v\in V \mbox{ with }v\ne 0\right\}. \end{align}$

Read more about this topic: Operator Norm

Famous quotes containing the words equivalent and/or definitions:

“Divorce is the psychological equivalent of a triple coronary bypass.”
—Mary Kay Blakely (20th century)

“Lord Byron is an exceedingly interesting person, and as such is it not to be regretted that he is a slave to the vilest and most vulgar prejudices, and as mad as the winds?
There have been many definitions of beauty in art. What is it? Beauty is what the untrained eyes consider abominable.”
—Edmond De Goncourt (1822–1896)