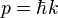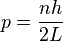Old Quantum Theory - de Broglie Waves

De Broglie Waves

In 1905, Einstein noted that the entropy of the quantized electromagnetic field oscillators in a box is, for short wavelength, equal to the entropy of a gas of point particles in the same box. The number of point particles is equal to the number of quanta. Einstein concluded that the quanta could be treated as if they were localizable objects (see page 139/140), particles of light, and named them photons.

Einstein's theoretical argument was based on thermodynamics, on counting the number of states, and so was not completely convincing. Nevertheless, he concluded that light had attributes of both waves and particles, more precisely that an electromagnetic standing wave with frequency with the quantized energy:

should be thought of as consisting of n photons each with an energy . Einstein could not describe how the photons were related to the wave.

The photons have momentum as well as energy, and the momentum had to be where is the wavenumber of the electromagnetic wave. This is required by relativity, because the momentum and energy form a four-vector, as do the frequency and wave-number.

In 1924, as a PhD candidate, Louis de Broglie proposed a new interpretation of the quantum condition. He suggested that all matter, electrons as well as photons, are described by waves obeying the relations.

or, expressed in terms of wavelength instead,

He then noted that the quantum condition:

counts the change in phase for the wave as it travels along the classical orbit, and requires that it be an integer multiple of . Expressed in wavelengths, the number of wavelengths along a classical orbit must be an integer. This is the condition for constructive interference, and it explained the reason for quantized orbits—the matter waves make standing waves only at discrete frequencies, at discrete energies.

For example, for a particle confined in a box, a standing wave must fit an integer number of wavelengths between twice the distance between the walls. The condition becomes:

so that the quantized momenta are:

reproducing the old quantum energy levels.

This development was given a more mathematical form by Einstein, who noted that the phase function for the waves: in a mechanical system should be identified with the solution to the Hamilton–Jacobi equation, an equation which even Hamilton considered to be the short-wavelength limit of wave mechanics.

These ideas led to the development of the Schrödinger equation.