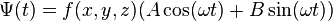Standing Waves
A standing wave is a continuous form of normal mode. In a standing wave, all the space elements (i.e. (x, y, z) coordinates) are oscillating in the same frequency and in phase (reaching the equilibrium point together), but each has a different amplitude.
The general form of a standing wave is:
where ƒ(x, y, z) represents the dependence of amplitude on location and the cosine\sine are the oscillations in time.
Physically, standing waves are formed by the interference (superposition) of waves and their reflections (although one may also say the opposite; that a moving wave is a superposition of standing waves). The geometric shape of the medium determines what would be the interference pattern, thus determines the ƒ(x, y, z) form of the standing wave. This space-dependence is called a normal mode.
Usually, for problems with continuous dependence on (x, y, z) there is no single or finite number of normal modes, but there are infinitely many normal modes. If the problem is bounded (i.e. it is defined on a finite section of space) there are countably many (a discrete infinity of ) normal modes (usually numbered n = 1, 2, 3, ...). If the problem is not bounded, there is a continuous spectrum of normal modes.
Read more about this topic: Normal Mode
Famous quotes containing the words standing and/or waves:
“If a person lost would conclude that after all he is not lost, he is not beside himself, but standing in his own old shoes on the very spot where he is, and that for the time being he will live there; but the places that have known him, they are lost,—how much anxiety and danger would vanish.”
—Henry David Thoreau (1817–1862)
“With these I would be.
And with water: the waves coming forward, without cessation,
The waves, altered by sand-bars, beds of kelp, miscellaneous
driftwood,
Topped by cross-winds, tugged at by sinuous undercurrents
The tide rustling in, sliding between the ridges of stone,
The tongues of water, creeping in, quietly.”
—Theodore Roethke (1908–1963)