Newtonian Dynamics - Newton's Second Law in A Multidimensional Space

Newton's Second Law in A Multidimensional Space

Let's consider particles with masses in the regular three-dimensional Euclidean space. Let be their radius-vectors in some inertial coordinate system. Then the motion of these particles is governed by Newton's second law applied to each of them

\frac{d\mathbf r_i}{dt}=\mathbf v_i,\qquad\frac{d\mathbf v_i}{dt}=\frac{\mathbf F_i(\mathbf r_1,\ldots,\mathbf r_N,\mathbf v_1,\ldots,\mathbf v_N,t)}{m_i},\quad i=1,\ldots,N.

(1)

The three-dimensional radius-vectors can be built into a single -dimensional radius-vector. Similarly, three-dimensional velocity vectors can be built into a single -dimensional velocity vector:

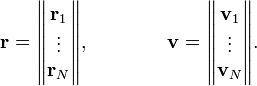
\mathbf r=\begin{Vmatrix} \mathbf r_1\\ \vdots\\ \mathbf r_N\end{Vmatrix},\qquad\qquad \mathbf v=\begin{Vmatrix} \mathbf v_1\\ \vdots\\ \mathbf v_N\end{Vmatrix}.

(2)

In terms of the multidimensional vectors (2) the equations (1) are written as

\frac{d\mathbf r}{dt}=\mathbf v,\qquad\frac{d\mathbf v}{dt}=\mathbf F(\mathbf r,\mathbf v,t),

(3)

i. e they take the form of Newton's second law applied to a single particle with the unit mass .

Definition. The equations (3) are called the equations of a Newtonian dynamical system in a flat multidimensional Euclidean space, which is called the configuration space of this system. Its points are marked by the radius-vector . The space whose points are marked by the pair of vectors is called the phase space of the dynamical system (3).

Read more about this topic:  Newtonian Dynamics

Famous quotes containing the words newton, law and/or space:

    “The next Augustan age will dawn on the other side of the Atlantic. There will, perhaps, be a Thucydides at Boston, a Xenophon at New York, and, in time, a Virgil at Mexico, and a Newton at Peru. At last, some curious traveller from Lima will visit England and give a description of the ruins of St. Paul’s, like the editions of Balbec and Palmyra.”
    —Horace Walpole (1717–1797)

    “The world is nothing, the man is all; in yourself is the law of all nature, and you know not yet how a globule of sap ascends; in yourself slumbers the whole of Reason; it is for you to know all, it is for you to dare all.”
    —Ralph Waldo Emerson (1803–1882)

    “This moment exhibits infinite space, but there is a space also wherein all moments are infinitely exhibited, and the everlasting duration of infinite space is another region and room of joys.”
    —Thomas Traherne (1636–1674)