Newtonian Dynamics - Embedding and The Induced Riemannian Metric

Embedding and The Induced Riemannian Metric

Geometrically, the vector-function (7) implements an embedding of the comfiguration space of the constrained Newtonian dynamical system into the -dimensional flat comfiguration space of the unconstrained Newtonian dynamical system (3). Due to this embedding the Euclidean structure of the ambient space induces the Riemannian metric onto the manifold . The components of the metric tensor of this induced metric are given by the formula

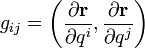\displaystyle g_{ij}=\left(\frac{\partial\mathbf r}{\partial q^i},\frac{\partial\mathbf r}{\partial q^j}\right) ,

(11)

where is the scalar product associated with the Euclidean structure (4).

Read more about this topic:  Newtonian Dynamics

Famous quotes containing the word induced:

    “It is a misfortune that necessity has induced men to accord greater license to this formidable engine, in order to obtain liberty, than can be borne with less important objects in view; for the press, like fire, is an excellent servant, but a terrible master.”
    —James Fenimore Cooper (1789–1851)