Constraints and Internal Coordinates
In some cases the motion of the particles with the masses can be constrained. Typical constraints look like scalar equations of the form
-
.
(5)
Constraints of the form (5) are called holonomic and stationary. In terms of the radius-vector of the Newtonian dynamical system (3) they are written as
-
.
(6)
Each such constraint reduces by one the number of degrees of freedom of the Newtonian dynamical system (3). Therefore the constrained system has degrees of freedom.
Definition. The constraint equations (6) define an -dimensional manifold within the configuration space of the Newtonian dynamical system (3). This manifold is called the configuration space of the constrained system. Its tangent bundle is called the phase space of the constrained system.
Let be the internal coordinates of a point of . Their usage is typical for the Lagrangian mechanics. The radius-vector is expressed as some definite function of :
-
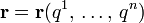.(7)
The vector-function (7) resolves the constraint equations (6) in the sense that upon substituting (7) into (6) the equations (6) are fulfilled identically in .
Read more about this topic: Newtonian Dynamics
Famous quotes containing the words constraints and/or internal:
“Play is a major avenue for learning to manage anxiety. It gives the child a safe space where she can experiment at will, suspending the rules and constraints of physical and social reality. In play, the child becomes master rather than subject.... Play allows the child to transcend passivity and to become the active doer of what happens around her.”
—Alicia F. Lieberman (20th century)
“When a person doesn’t understand something, he feels internal discord: however he doesn’t search for that discord in himself, as he should, but searches outside of himself. Thence a war develops with that which he doesn’t understand.”
—Anton Pavlovich Chekhov (1860–1904)