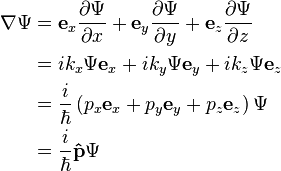Momentum Operator - Origin From de Broglie Plane Waves

Origin From De Broglie Plane Waves

The momentum and energy operators can be constructed in the following way.

One dimension

Starting in one dimension, using the plane wave solution to Schrödinger's equation:

The first order partial derivative with respect to space is

By expressing of k from the De Broglie relation:

the formula for the derivative of ψ becomes:

This suggests the operator equivalence:

so the momentum value p is a scalar factor, the momentum of the particle and the value that is measured, is the eigenvalue of the operator.

Since the partial derivative is a linear operator, the momentum operator is also linear, and because any wavefunction can be expressed as a superposition of other states, when this momentum operator acts on the entire superimposed wave, it yields the momentum eigenvalues for each plane wave component, the momenta add to the total momentum of the superimposed wave.

Three dimensions

The derivation in three dimensions is the same, except using the gradient operator del is used instead of one partial derivative. In three dimensions, the plane wave solution to Schrödinger's equation is:

and the gradient is

\begin{align} \nabla \Psi & = \bold{e}_x\frac{\partial \Psi}{\partial x} + \bold{e}_y\frac{\partial \Psi}{\partial y} + \bold{e}_z\frac{\partial \Psi}{\partial z} \\ & = i k_x\Psi\bold{e}_x + i k_y\Psi\bold{e}_y+ i k_z\Psi\bold{e}_z \\ & = \frac{i}{\hbar} \left ( p_x\bold{e}_x + p_y\bold{e}_y+ p_z\bold{e}_z \right)\Psi \\ & = \frac{i}{\hbar} \bold{\hat{p}}\Psi \\ \end{align} \,\!

where, and are the unit vectors for the three spatial dimensions, hence

This momentum operator is in position space because the partial derivatives were taken with respect to the spatial variables.

Read more about this topic:  Momentum Operator

Famous quotes containing the words origin, plane and/or waves:

    “There are certain books in the world which every searcher for truth must know: the Bible, the Critique of Pure Reason, the Origin of Species, and Karl Marx’s Capital.”
    —W.E.B. (William Edward Burghardt)

    “As for the dispute about solitude and society, any comparison is impertinent. It is an idling down on the plane at the base of a mountain, instead of climbing steadily to its top.”
    —Henry David Thoreau (1817–1862)

    “What is Americanism? Every one has a different answer. Some people say it is never to submit to the dictation of a King. Others say Americanism is the pride of liberty and the defence of an insult to the flag with their gore. When some half-developed person tramples on that flag, we should be ready to pour out the blood of the nation, they say. But do we not sit in silence when that flag waves over living conditions which should be an insult to all patriotism?”
    —Anna Howard Shaw (1847–1919)