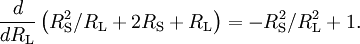Maximum Power Transfer Theorem - Calculus-based Proof For Purely Resistive Circuits

Calculus-based Proof For Purely Resistive Circuits

(See Cartwright for a non-calculus-based proof)

In the diagram opposite, power is being transferred from the source, with voltage and fixed source resistance, to a load with resistance, resulting in a current . By Ohm's law, is simply the source voltage divided by the total circuit resistance:

I = {V \over R_\mathrm{S} + R_\mathrm{L}}. \,\!

The power dissipated in the load is the square of the current multiplied by the resistance:

P_\mathrm{L} = I^2 R_\mathrm{L} = {{\left( {V \over {R_\mathrm{S} + R_\mathrm{L}}} \right) }^2} R_\mathrm{L} = {{V^2} \over {R_\mathrm{S}^2 / R_\mathrm{L} + 2R_\mathrm{S} + R_\mathrm{L}}}. \,\!

The value of for which this expression is a maximum could be calculated by differentiating it, but it is easier to calculate the value of for which the denominator

R_\mathrm{S}^2 / R_\mathrm{L} + 2R_\mathrm{S} + R_\mathrm{L} \,\!

is a minimum. The result will be the same in either case. Differentiating the denominator with respect to :

{d\over{dR_\mathrm{L}}} \left( {R_\mathrm{S}^2 / R_\mathrm{L} + 2R_\mathrm{S} + R_\mathrm{L}} \right) = -R_\mathrm{S}^2 / R_\mathrm{L}^2+1. \,\!

For a maximum or minimum, the first derivative is zero, so

{R_\mathrm{S}^2 / R_\mathrm{L}^2} = 1 \,\!

or

In practical resistive circuits, and are both positive, so the positive sign in the above is the correct solution. To find out whether this solution is a minimum or a maximum, the denominator expression is differentiated again:

This is always positive for positive values of and, showing that the denominator is a minimum, and the power is therefore a maximum, when

A note of caution is in order here. This last statement, as written, implies to many people that for a given load, the source resistance must be set equal to the load resistance for maximum power transfer. However, this equation only applies if the source resistance cannot be adjusted, e.g., with antennas (see the first line in the proof stating "fixed source resistance"). For any given load resistance a source resistance of zero is the way to transfer maximum power to the load. As an example, a 100 volt source with an internal resistance of 10 ohms connected to a 10 ohm load will deliver 250 watts to that load. Make the source resistance zero ohms and the load power jumps to 1000 watts.

Read more about this topic:  Maximum Power Transfer Theorem

Famous quotes containing the words proof, purely and/or circuits:

    “The proof of a poet is that his country absorbs him as affectionately as he has absorbed it.”
    —Walt Whitman (1819–1892)

    “Every man is the builder of a temple, called his body, to the god he worships, after a style purely his own, nor can he get off by hammering marble instead. We are all sculptors and painters, and our material is our own flesh and bones. Any nobleness begins at once to refine a man’s features, any meanness or sensuality to imbrute them.”
    —Henry David Thoreau (1817–1862)

    “The Buddha, the Godhead, resides quite as comfortably in the circuits of a digital computer or the gears of a cycle transmission as he does at the top of a mountain or in the petals of a flower.”
    —Robert M. Pirsig (b. 1928)