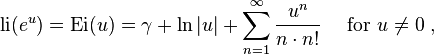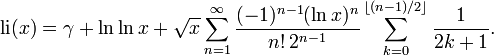Logarithmic Integral Function - Series Representation

Series Representation

The function li(x) is related to the exponential integral Ei(x) via the equation

which is valid for x > 0. This identity provides a series representation of li(x) as

${\rm li} (e^u) = \hbox{Ei}(u) = \gamma + \ln |u| + \sum_{n=1}^\infty {u^{n}\over n \cdot n!} \quad \text{ for } u \ne 0 \;,$

where γ ≈ 0.57721 56649 01532 ... is the Euler–Mascheroni gamma constant. A more rapidly convergent series due to Ramanujan is

${\rm li} (x) = \gamma + \ln \ln x + \sqrt{x} \sum_{n=1}^\infty \frac{ (-1)^{n-1} (\ln x)^n} {n! \, 2^{n-1}} \sum_{k=0}^{\lfloor (n-1)/2 \rfloor} \frac{1}{2k+1} .$

Read more about this topic: Logarithmic Integral Function

Famous quotes containing the word series:

“The woman’s world ... is shown as a series of limited spaces, with the woman struggling to get free of them. The struggle is what the film is about; what is struggled against is the limited space itself. Consequently, to make its point, the film has to deny itself and suggest it was the struggle that was wrong, not the space.”
—Jeanine Basinger (b. 1936)