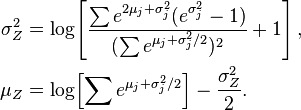Log-normal Distribution - Related Distributions

Related Distributions

  • If is a normal distribution, then
  • If is distributed log-normally, then is a normal random variable.
  • If are n independent log-normally distributed variables, and, then Y is also distributed log-normally:
  • Let be independent log-normally distributed variables with possibly varying σ and μ parameters, and . The distribution of Y has no closed-form expression, but can be reasonably approximated by another log-normal distribution Z at the right tail. Its probability density function at the neighborhood of 0 has been characterized and it does not resemble any log-normal distribution. A commonly used approximation (due to Fenton and Wilkinson) is obtained by matching the mean and variance:
\begin{align} \sigma^2_Z &= \log\!\left, \\ \mu_Z &= \log\!\left - \frac{\sigma^2_Z}{2}. \end{align}

In the case that all have the same variance parameter, these formulas simplify to

\begin{align} \sigma^2_Z &= \log\!\left, \\ \mu_Z &= \log\!\left + \frac{\sigma^2}{2} - \frac{\sigma^2_Z}{2}. \end{align}
  • If, then X + c is said to have a shifted log-normal distribution with support x ∈ (c, +∞). E = E + c, Var = Var.
  • If, then
  • If, then
  • If then for
  • Lognormal distribution is a special case of semi-bounded Johnson distribution
  • If with, then (Suzuki distribution)

Read more about this topic:  Log-normal Distribution

Famous quotes containing the word related:

    “One does not realize the historical sensation as a re-experiencing, but as an understanding that is closely related to the understanding of music, or rather of the world by means of music.”
    —Johan Huizinga (1872–1945)