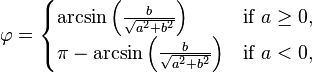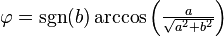List of Trigonometric Identities - Linear Combinations

Linear Combinations

For some purposes it is important to know that any linear combination of sine waves of the same period or frequency but different phase shifts is also a sine wave with the same period or frequency, but a different phase shift. In the case of a non-zero linear combination of a sine and cosine wave (which is just a sine wave with a phase shift of π/2), we have

where

$\varphi = \begin{cases}\arcsin \left(\frac{b}{\sqrt{a^2+b^2}}\right) & \text{if }a \ge 0, \\ \pi-\arcsin \left(\frac{b}{\sqrt{a^2+b^2}}\right) & \text{if }a < 0, \end{cases}$

or equivalently

$\varphi = \text{sgn}(b)\arccos \left(\tfrac{a}{\sqrt{a^2+b^2}}\right)$

or even

$\varphi = \arctan \left(\frac{b}{a}\right) + \begin{cases} 0 & \text{if }a \ge 0, \\ \pi & \text{if }a < 0, \end{cases}$

or using the atan2 function

More generally, for an arbitrary phase shift, we have

where

and

$\beta = \arctan \left(\frac{b\sin \alpha}{a + b\cos \alpha}\right) + \begin{cases} 0 & \text{if } a + b\cos \alpha \ge 0, \\ \pi & \text{if } a + b\cos \alpha < 0. \end{cases}$

The general case reads

where

and

Famous quotes containing the word combinations:

“You should try to understand every thing you see and hear; to act and judge for yourselves; to remember you each have a soul of your own to account for; M a mind of your own to improve. When you once get these ideas fixed, and learn to act upon them, no man or set of men, no laws, customs, or combinations of them can seriously oppress you.”
—Jane Grey Swisshelm (1815–1884)