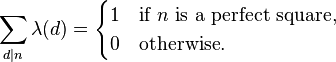The Liouville function, denoted by λ(n) and named after Joseph Liouville, is an important function in number theory.
If n is a positive integer, then λ(n) is defined as:
where Ω(n) is the number of prime factors of n, counted with multiplicity (sequence A008836 in OEIS).
λ is completely multiplicative since Ω(n) is completely additive. The number one has no prime factors, so Ω(1) = 0 and therefore λ(1) = 1. The Liouville function satisfies the identity:
The Liouville function's Dirichlet inverse is the absolute value of the Mobius function.
Read more about Liouville Function: Series, Conjectures
Famous quotes containing the word function:
“Philosophical questions are not by their nature insoluble. They are, indeed, radically different from scientific questions, because they concern the implications and other interrelations of ideas, not the order of physical events; their answers are interpretations instead of factual reports, and their function is to increase not our knowledge of nature, but our understanding of what we know.”
—Susanne K. Langer (1895–1985)