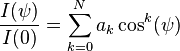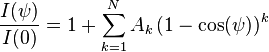Limb Darkening - Calculation of Limb Darkening

Calculation of Limb Darkening

In the figure on the right, as long as the observer at point P is outside the stellar atmosphere, the intensity seen in the direction θ will be a function only of the angle of incidence ψ. This is most conveniently approximated as a polynomial in cos(ψ)

where I(ψ) is the intensity seen at P along a line of sight forming angle ψ with respect to the stellar radius, and I(0) is the central intensity. In order that the ratio be unity for ψ=0, we must have:

For example, for a Lambertian radiator (no limb darkening) we will have all a_k=0 except a₀=1. As another example, for the sun at 550 nm, the limb darkening is well expressed by N=2 and

(See Cox, 2000). Note - the equation for limb darkening is sometimes more conveniently written as:

which now has N independent coefficients rather than N+1 coefficients which must sum to unity.

We can convert from ψ to θ using the relationship:

where Ω is the angle from the observer to the limb of the star.

The above approximation can be used to derive an analytic expression for the ratio of the mean intensity to the central intensity. The mean intensity I_m is the integral of the intensity over the disk of the star divided by the solid angle subtended by the disk:

where dω=sin(θ)dθdφ is a solid angle element and the integrals are over the disk: 0≤φ≤2π and 0≤θ≤Ω. Although this equation can be solved analytically, it is rather cumbersome. However, for an observer at infinite distance from the star, the above equation simplifies to: