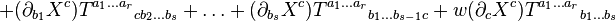Lie Derivative - Coordinate Expressions

Coordinate Expressions

In local coordinate notation, for a type (r,s) tensor field, the Lie derivative along is

\begin{align} (\mathcal{L}_X T) ^{a_1 \ldots a_r}{}_{b_1 \ldots b_s} = & X^c(\partial_c T^{a_1 \ldots a_r}{}_{b_1 \ldots b_s}) \\ & - (\partial_c X ^{a_1}) T ^{c a_2 \ldots a_r}{}_{b_1 \ldots b_s} - \ldots - (\partial_c X^{a_r}) T ^{a_1 \ldots a_{r-1}c}{}_{b_1 \ldots b_s} \\ & + (\partial_{b_1} X^c) T ^{a_1 \ldots a_r}{}_{c b_2 \ldots b_s} + \ldots + (\partial_{b_s}X^c) T ^{a_1 \ldots a_r}{}_{b_1 \ldots b_{s-1} c} \end{align}

here, the notation means taking the partial derivative with respect to the coordinate . Alternatively, if we are using a torsion-free connection (e.g. the Levi Civita connection), then the partial derivative can be replaced with the covariant derivative . The Lie derivative of a tensor is another tensor of the same type, i.e. even though the individual terms in the expression depend on the choice of coordinate system, the expression as a whole results in a tensor

which is independent of any coordinate system.

The definition can be extended further to tensor densities of weight w for any real w. If T is such a tensor density, then its Lie derivative is a tensor density of the same type and weight.

+ (\partial_{b_1} X^c) T ^{a_1 \ldots a_r}{}_{c b_2 \ldots b_s} + \ldots + (\partial_{b_s} X^c) T ^{a_1 \ldots a_r}{}_{b_1 \ldots b_{s-1} c} + w (\partial_{c} X^c) T ^{a_1 \ldots a_r}{}_{b_1 \ldots b_{s}}

Notice the new term at the end of the expression.

Read more about this topic:  Lie Derivative

Famous quotes containing the word expressions:

    “Its idea of “production value” is spending a million dollars dressing up a story that any good writer would throw away. Its vision of the rewarding movie is a vehicle for some glamour-puss with two expressions and eighteen changes of costume, or for some male idol of the muddled millions with a permanent hangover, six worn-out acting tricks, the build of a lifeguard, and the mentality of a chicken-strangler.”
    —Raymond Chandler (1888–1959)