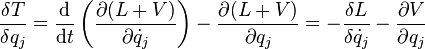Lagrangian Mechanics - Lagrange Equations of The First Kind

Lagrange Equations of The First Kind

Lagrange introduced an analytical method for finding stationary points using the method of Lagrange multipliers, and also applied it to mechanics.

For a system subject to the constraint equation on the generalized coordinates:

where A is a constant, then Lagrange's equations of the first kind are:

where λ is the Lagrange multiplier. By analogy with the mathematical procedure, we can write:

where

denotes the variational derivative.

For e constraint equations F1, F2,..., Fe, there is a Lagrange multiplier for each constraint equation, and Lagrange's equations of the first kind generalize to:

Lagrange's equations (1st kind)

This procedure does increase the number of equations, but there are enough to solve for all of the multipliers. The number of equations generated is the number of constraint equations plus the number of coordinates, i.e. e + m. The advantage of the method is that (potentially complicated) substitution and elimination of variables linked by constraint equations can be bypassed.

There is a connection between the constraint equations Fj and the constraint forces Nj acting in the conservative system (forces are conservative):

which is derived below.

Derivation of connection between constraint equations and forces
The generalized constraint forces are given by (using the definition of generalized force above):

and using the kinetic energy equation of motion (blue box above):

For conservative systems (see below)

so

\frac{\delta T}{\delta q_j} = \sum_{i=1}^n \mathbf{F}_i\cdot\frac{\partial \mathbf{r}_i}{\partial q_j} =\sum_{i=1}^n (-\nabla V_i + \mathbf{N}_i)\cdot\frac{\partial \mathbf{r}_i}{\partial q_j} =-\sum_{i=1}^n\nabla V_i\cdot\frac{\partial \mathbf{r}_i}{\partial q_j}+\sum_{i=1}^n \mathbf{N}_i\cdot\frac{\partial \mathbf{r}_i}{\partial q_j} =-\frac{\partial V}{\partial q_j} + N_j

and

\frac{\delta T}{\delta q_j}=\frac{\mathrm{d}}{\mathrm{d}t} \left ( \frac{\partial (L+V)}{\partial \dot{q}_j} \right ) - \frac {\partial (L+V)}{\partial q_j} =-\frac{\delta L}{\delta \dot{q}_j} - \frac {\partial V}{\partial q_j}

equating leads to

and finally equating to Lagrange's equations of the first kind implies:

So each constraint equation corresponds to a constraint force (in a conservative system).

Read more about this topic:  Lagrangian Mechanics

Famous quotes containing the word kind:

    “Fifty years from now, it will not matter what kind of car you drove, what kind of house you lived in, how much you had in your bank account, or what your clothes looked like, But the world may be a little better because you were important in the life of a child.”
    —Anonymous. Quoted in The Winning Family, by Louise Hart, ch. 1 (1987)