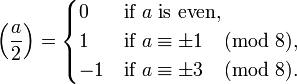Definition
Let n be a non-zero integer, with prime factorization
where u is a unit (i.e., u is 1 or −1), and the pi are primes. Let a be an integer. The Kronecker symbol (a|n) is defined by
For odd pi, the number (a|pi) is simply the usual Legendre symbol. This leaves the case when pi = 2. We define (a|2) by
Since it extends the Jacobi symbol, the quantity (a|u) is simply 1 when u = 1. When u = −1, we define it by
Finally, we put
These extensions suffice to define the Kronecker symbol for all integer values n.
Read more about this topic: Kronecker Symbol
Famous quotes containing the word definition:
“Mothers often are too easily intimidated by their children’s negative reactions...When the child cries or is unhappy, the mother reads this as meaning that she is a failure. This is why it is so important for a mother to know...that the process of growing up involves by definition things that her child is not going to like. Her job is not to create a bed of roses, but to help him learn how to pick his way through the thorns.”
—Elaine Heffner (20th century)
“... we all know the wag’s definition of a philanthropist: a man whose charity increases directly as the square of the distance.”
—George Eliot [Mary Ann (or Marian)
“I’m beginning to think that the proper definition of “Man” is “an animal that writes letters.””
—Lewis Carroll [Charles Lutwidge Dodgson] (1832–1898)