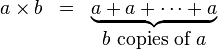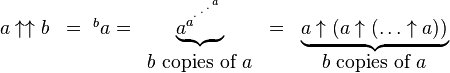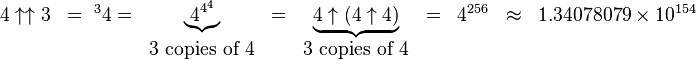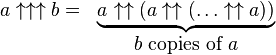Knuth's Up-arrow Notation - Introduction

Introduction

The ordinary arithmetical operations of addition, multiplication and exponentiation are naturally extended into a sequence of hyperoperations as follows.

Multiplication by a natural number is defined as iterated addition:

\begin{matrix} a\times b & = & \underbrace{a+a+\dots+a} \\ & & b\mbox{ copies of }a \end{matrix}

For example,

\begin{matrix} 4\times 3 & = & \underbrace{4+4+4} & = & 12\\ & & 3\mbox{ copies of }4 \end{matrix}

Exponentiation for a natural power is defined as iterated multiplication, which Knuth denoted by a single up-arrow:

\begin{matrix} a\uparrow b= a^b = & \underbrace{a\times a\times\dots\times a}\\ & b\mbox{ copies of }a \end{matrix}

For example,

\begin{matrix} 4\uparrow 3= 4^3 = & \underbrace{4\times 4\times 4} & = & 64\\ & 3\mbox{ copies of }4 \end{matrix}

To extend the sequence of operations beyond exponentiation, Knuth defined a “double arrow” operator to denote iterated exponentiation (tetration):

\begin{matrix} a\uparrow\uparrow b & = {\ ^{b}a} = & \underbrace{a^{a^{{}^{.\,^{.\,^{.\,^a}}}}}} & = & \underbrace{a\uparrow (a\uparrow(\dots\uparrow a))} \\ & & b\mbox{ copies of }a & & b\mbox{ copies of }a \end{matrix}

For example,

\begin{matrix} 4\uparrow\uparrow 3 & = {\ ^{3}4} = & \underbrace{4^{4^4}} & = & \underbrace{4\uparrow (4\uparrow 4)} & = & 4^{256} & \approx & 1.34078079\times 10^{154}& \\ & & 3\mbox{ copies of }4 & & 3\mbox{ copies of }4 \end{matrix}

Here and below evaluation is to take place from right to left, as Knuth's arrow operators (just like exponentiation) are defined to be right-associative.

According to this definition,

etc.

This already leads to some fairly large numbers, but Knuth extended the notation. He went on to define a “triple arrow” operator for iterated application of the “double arrow” operator (also known as pentation):

\begin{matrix} a\uparrow\uparrow\uparrow b= & \underbrace{a_{}\uparrow\uparrow (a\uparrow\uparrow(\dots\uparrow\uparrow a))}\\ & b\mbox{ copies of }a \end{matrix}

followed by a 'quadruple arrow' operator (also known as hexation):

\begin{matrix} a\uparrow\uparrow\uparrow\uparrow b= & \underbrace{a_{}\uparrow\uparrow\uparrow (a\uparrow\uparrow\uparrow(\dots\uparrow\uparrow\uparrow a))}\\ & b\mbox{ copies of }a \end{matrix}

and so on. The general rule is that an -arrow operator expands into a right-associative series of -arrow operators. Symbolically,

\begin{matrix} a\ \underbrace{\uparrow_{}\uparrow\!\!\dots\!\!\uparrow}_{n}\ b= \underbrace{a\ \underbrace{\uparrow\!\!\dots\!\!\uparrow}_{n-1} \ (a\ \underbrace{\uparrow_{}\!\!\dots\!\!\uparrow}_{n-1} \ (\dots \ \underbrace{\uparrow_{}\!\!\dots\!\!\uparrow}_{n-1} \ a))}_{b\text{ copies of }a} \end{matrix}

Examples:

\begin{matrix} 3\uparrow\uparrow\uparrow3 = 3\uparrow\uparrow3\uparrow\uparrow3 = 3\uparrow\uparrow(3\uparrow3\uparrow3) = & \underbrace{3_{}\uparrow 3\uparrow\dots\uparrow 3} \\ & 3\uparrow3\uparrow3\mbox{ copies of }3 \end{matrix} \begin{matrix} = & \underbrace{3_{}\uparrow 3\uparrow\dots\uparrow 3} \\ & \mbox{7,625,597,484,987 copies of 3} \end{matrix}

The notation is commonly used to denote with n arrows.

Read more about this topic:  Knuth's Up-arrow Notation

Famous quotes containing the word introduction:

    “Such is oftenest the young man’s introduction to the forest, and the most original part of himself. He goes thither at first as a hunter and fisher, until at last, if he has the seeds of a better life in him, he distinguishes his proper objects, as a poet or naturalist it may be, and leaves the gun and fish-pole behind. The mass of men are still and always young in this respect.”
    —Henry David Thoreau (1817–1862)

    “For better or worse, stepparenting is self-conscious parenting. You’re damned if you do, and damned if you don’t.”
    —Anonymous Parent. Making It as a Stepparent, by Claire Berman, introduction (1980, repr. 1986)

    “My objection to Liberalism is this—that it is the introduction into the practical business of life of the highest kind—namely, politics—of philosophical ideas instead of political principles.”
    —Benjamin Disraeli (1804–1881)