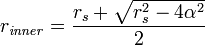Kerr Metric - Important Surfaces

Important Surfaces

The Kerr metric has two physical relevant surfaces on which it appears to be singular. The inner surface corresponds to an event horizon similar to that observed in the Schwarzschild metric; this occurs where the purely radial component grr of the metric goes to infinity. Solving the quadratic equation 1/grr = 0 yields the solution:

r_\mathit{inner} = \frac{r_{s} + \sqrt{r_{s}^{2} - 4\alpha^{2}}}{2}

Another singularity occurs where the purely temporal component gtt of the metric changes sign from positive to negative. Again solving a quadratic equation gtt=0 yields the solution:

r_\mathit{outer} = \frac{r_{s} + \sqrt{r_{s}^{2} - 4\alpha^{2} \cos^{2}\theta}}{2}

Due to the cos2θ term in the square root, this outer surface resembles a flattened sphere that touches the inner surface at the poles of the rotation axis, where the colatitude θ equals 0 or π; the space between these two surfaces is called the ergosphere. There are two other solutions to these quadratic equations, but they lie within the event horizon, where the Kerr metric is not used, since it has unphysical properties (see below).

A moving particle experiences a positive proper time along its worldline, its path through spacetime. However, this is impossible within the ergosphere, where gtt is negative, unless the particle is co-rotating with the interior mass M with an angular speed at least of Ω. Thus, no particle can rotate opposite to the central mass within the ergosphere.

As with the event horizon in the Schwarzschild metric the apparent singularities at rinner and router are an illusion created by the choice of coordinates (i.e., they are coordinate singularities). In fact, the space-time can be smoothly continued through them by an appropriate choice of coordinates.

Read more about this topic:  Kerr Metric

Famous quotes containing the words important and/or surfaces:

    “The growing of food and the growing of children are both vital to the family’s survival.... Who would dare make the judgment that holding your youngest baby on your lap is less important than weeding a few more yards in the maize field? Yet this is the judgment our society makes constantly. Production of autos, canned soup, advertising copy is important. Housework—cleaning, feeding, and caring—is unimportant.”
    —Debbie Taylor (20th century)

    “But ice-crunching and loud gum-chewing, together with drumming on tables, and whistling the same tune seventy times in succession, because they indicate an indifference on the part of the perpetrator to the rest of the world in general, are not only registered on the delicate surfaces of the brain but eat little holes in it until it finally collapses or blows up.”
    —Robert Benchley (1889–1945)