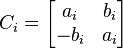Jordan Normal Form - Real Matrices

Real Matrices

If A is a real matrix, its Jordan form can still be non-real, however there exists a real invertible matrix P such that P-1AP = J is a real block diagonal matrix with each block being a real Jordan block. A real Jordan block is either identical to a complex Jordan block (if the corresponding eigenvalue is real), or is a block matrix itself, consisting of 2×2 blocks as follows (for non-real eigenvalue ). The diagonal blocks are identical, of the form

C_i = \begin{bmatrix} a_i & b_i \\ -b_i & a_i \\ \end{bmatrix}

and describe multiplication by in the complex plane. The superdiagonal blocks are 2×2 identity matrices. The full real Jordan block is given by

J_i = \begin{bmatrix} C_i & I & \; & \; \\ \; & C_i & \ddots & \; \\ \; & \; & \ddots & I \\ \; & \; & \; & C_i \\ \end{bmatrix}.

This real Jordan form is a consequence of the complex Jordan form. For a real matrix the nonreal eigenvectors and generalized eigenvectors can always be chosen to form complex conjugate pairs. Taking the real and imaginary part (linear combination of the vector and its conjugate), the matrix has this form in the new basis.

Read more about this topic:  Jordan Normal Form

Famous quotes containing the word real:

    “The real risks for any artist are taken ... in pushing the work to the limits of what is possible, in the attempt to increase the sum of what it is possible to think. Books become good when they go to this edge and risk falling over it—when they endanger the artist by reason of what he has, or has not, artistically dared.”
    —Salman Rushdie (b. 1947)