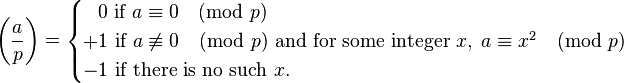Jacobi Symbol - Definition

Definition

For any integer a and any positive odd integer n the Jacobi symbol is defined as the product of the Legendre symbols corresponding to the prime factors of n:


represents the Legendre symbol, defined for all integers a and all odd primes p by

\left(\frac{a}{p}\right) = \begin{cases} \;\;\,0\mbox{ if } a \equiv 0 \pmod{p} \\+1\mbox{ if }a \not\equiv 0\pmod{p} \mbox{ and for some integer }x, \;a\equiv x^2\pmod{p} \\-1\mbox{ if there is no such } x. \end{cases}

Following the normal convention for the empty product, The Legendre and Jacobi symbols are indistinguishable exactly when the lower argument is an odd prime, in which case they have the same value.

Read more about this topic:  Jacobi Symbol

Famous quotes containing the word definition:

    “It is very hard to give a just definition of love. The most we can say of it is this: that in the soul, it is a desire to rule; in the spirit, it is a sympathy; and in the body, it is but a hidden and subtle desire to possess—after many mysteries—what one loves.”
    —François, Duc De La Rochefoucauld (1613–1680)

    “Scientific method is the way to truth, but it affords, even in
    principle, no unique definition of truth. Any so-called pragmatic
    definition of truth is doomed to failure equally.”
    —Willard Van Orman Quine (b. 1908)

    “The definition of good prose is proper words in their proper places; of good verse, the most proper words in their proper places. The propriety is in either case relative. The words in prose ought to express the intended meaning, and no more; if they attract attention to themselves, it is, in general, a fault.”
    —Samuel Taylor Coleridge (1772–1834)