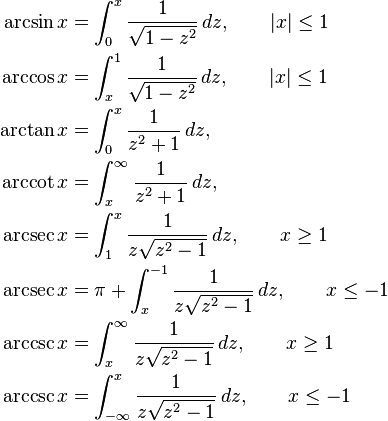Inverse Trigonometric Functions - Expression As Definite Integrals

Expression As Definite Integrals

Integrating the derivative and fixing the value at one point gives an expression for the inverse trigonometric function as a definite integral:

\begin{align} \arcsin x &{}= \int_0^x \frac {1} {\sqrt{1 - z^2}}\,dz,\qquad |x| \leq 1\\ \arccos x &{}= \int_x^1 \frac {1} {\sqrt{1 - z^2}}\,dz,\qquad |x| \leq 1\\ \arctan x &{}= \int_0^x \frac 1 {z^2 + 1}\,dz,\\ \arccot x &{}= \int_x^\infty \frac {1} {z^2 + 1}\,dz,\\ \arcsec x &{}= \int_1^x \frac 1 {z \sqrt{z^2 - 1}}\,dz, \qquad x \geq 1\\ \arcsec x &{}= \pi + \int_x^{-1} \frac 1 {z \sqrt{z^2 - 1}}\,dz, \qquad x \leq -1\\ \arccsc x &{}= \int_x^\infty \frac {1} {z \sqrt{z^2 - 1}}\,dz, \qquad x \geq 1\\ \arccsc x &{}= \int_{-\infty}^x \frac {1} {z \sqrt{z^2 - 1}}\,dz, \qquad x \leq -1 \end{align}

When x equals 1, the integrals with limited domains are improper integrals, but still well-defined.

Read more about this topic:  Inverse Trigonometric Functions

Famous quotes containing the words expression and/or definite:

    “I have always looked upon decay as being just as wonderful and rich an expression of life as growth.”
    —Henry Miller (1891–1980)

    “The artistic temperament is a disease that affects amateurs.... Artists of a large and wholesome vitality get rid of their art easily, as they breathe easily or perspire easily. But in artists of less force, the thing becomes a pressure, and produces a definite pain, which is called the artistic temperament.”
    —Gilbert Keith Chesterton (1874–1936)