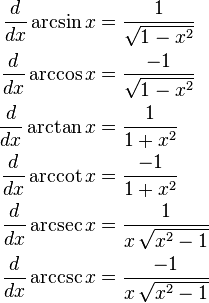Inverse Trigonometric Functions - Derivatives of Inverse Trigonometric Functions

Derivatives of Inverse Trigonometric Functions

Simple derivatives for real and complex values of x are as follows:

\begin{align} \frac{d}{dx} \arcsin x & {}= \frac{1}{\sqrt{1-x^2}}\\ \frac{d}{dx} \arccos x & {}= \frac{-1}{\sqrt{1-x^2}}\\ \frac{d}{dx} \arctan x & {}= \frac{1}{1+x^2}\\ \frac{d}{dx} \arccot x & {}= \frac{-1}{1+x^2}\\ \frac{d}{dx} \arcsec x & {}= \frac{1}{x\,\sqrt{x^2-1}}\\ \frac{d}{dx} \arccsc x & {}= \frac{-1}{x\,\sqrt{x^2-1}} \end{align}

Only for real values of x:

\begin{align} \frac{d}{dx} \arcsec x & {}= \frac{1}{|x|\,\sqrt{x^2-1}}; \qquad |x| > 1\\ \frac{d}{dx} \arccsc x & {}= \frac{-1}{|x|\,\sqrt{x^2-1}}; \qquad |x| > 1 \end{align}

For a sample derivation: if, we get:

Read more about this topic:  Inverse Trigonometric Functions

Famous quotes containing the words inverse and/or functions:

    “The quality of moral behaviour varies in inverse ratio to the number of human beings involved.”
    —Aldous Huxley (1894–1963)

    “The mind is a finer body, and resumes its functions of feeding, digesting, absorbing, excluding, and generating, in a new and ethereal element. Here, in the brain, is all the process of alimentation repeated, in the acquiring, comparing, digesting, and assimilating of experience. Here again is the mystery of generation repeated.”
    —Ralph Waldo Emerson (1803–1882)