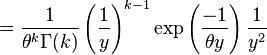Inverse-gamma Distribution - Derivation From Gamma Distribution

Derivation From Gamma Distribution

The pdf of the gamma distribution is

and define the transformation then the resulting transformation is

$f_Y(y) = f_X \left( g^{-1}(y) \right) \left| \frac{d}{dy} g^{-1}(y) \right|$

$= \frac{1}{\theta^k \Gamma(k)} \left( \frac{1}{y} \right)^{k-1} \exp \left( \frac{-1}{\theta y} \right) \frac{1}{y^2}$

$= \frac{1}{\theta^k \Gamma(k)} \left( \frac{1}{y} \right)^{k+1} \exp \left( \frac{-1}{\theta y} \right)$

$= \frac{1}{\theta^k \Gamma(k)} y^{-k-1} \exp \left( \frac{-1}{\theta y} \right).$

Replacing with ; with ; and with results in the inverse-gamma pdf shown above

$f(x) = \frac{\beta^\alpha}{\Gamma(\alpha)} x^{-\alpha-1} \exp \left( \frac{-\beta}{x} \right).$

Read more about this topic: Inverse-gamma Distribution

Famous quotes containing the word distribution:

“The man who pretends that the distribution of income in this country reflects the distribution of ability or character is an ignoramus. The man who says that it could by any possible political device be made to do so is an unpractical visionary. But the man who says that it ought to do so is something worse than an ignoramous and more disastrous than a visionary: he is, in the profoundest Scriptural sense of the word, a fool.”
—George Bernard Shaw (1856–1950)