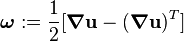Infinitesimal Strain Theory - Infinitesimal Rotation Tensor

Infinitesimal Rotation Tensor

The infinitesimal strain tensor is defined as

$\boldsymbol{\varepsilon} = \frac{1}{2}$

Therefore the displacement gradient can be expressed as

$\boldsymbol{\nabla}\mathbf{u} = \boldsymbol{\varepsilon} + \boldsymbol{\omega}$

where

$\boldsymbol{\omega} := \frac{1}{2}$

The quantity is the infinitesimal rotation tensor. This tensor is skew symmetric. For infinitesimal deformations the scalar components of satisfy the condition . Note that the displacement gradient is small only if both the strain tensor and the rotation tensor are infinitesimal.

Read more about this topic: Infinitesimal Strain Theory

Famous quotes containing the word rotation:

“The lazy manage to keep up with the earth’s rotation just as well as the industrious.”
—Mason Cooley (b. 1927)