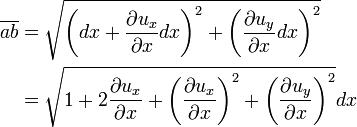Infinitesimal Strain Theory - Geometric Derivation of The Infinitesimal Strain Tensor

Geometric Derivation of The Infinitesimal Strain Tensor

Considering a two-dimensional deformation of an infinitesimal rectangular material element with dimensions by (Figure 1), which after deformation, takes the form of a rhombus. From the geometry of Figure 1 we have

\begin{align} \overline {ab} &= \sqrt{\left(dx+\frac{\partial u_x}{\partial x}dx \right)^2 + \left( \frac{\partial u_y}{\partial x}dx \right)^2} \\ &= \sqrt{1+2\frac{\partial u_x}{\partial x}+\left(\frac{\partial u_x}{\partial x}\right)^2 + \left(\frac{\partial u_y}{\partial x}\right)^2}dx \\ \end{align}\,\!

For very small displacement gradients, i.e., we have

The normal strain in the -direction of the rectangular element is defined by

and knowing that, we have

Similarly, the normal strain in the -direction, and -direction, becomes

The engineering shear strain, or the change in angle between two originally orthogonal material lines, in this case line and, is defined as

From the geometry of Figure 1 we have

For small rotations, i.e. and are we have

and, again, for small displacement gradients, we have

thus

By interchanging and and and, it can be shown that

Similarly, for the - and - planes, we have

It can be seen that the tensorial shear strain components of the infinitesimal strain tensor can then be expressed using the engineering strain definition, as

\left[\begin{matrix} \varepsilon_{xx} & \varepsilon_{xy} & \varepsilon_{xz} \\ \varepsilon_{yx} & \varepsilon_{yy} & \varepsilon_{yz} \\ \varepsilon_{zx} & \varepsilon_{zy} & \varepsilon_{zz} \\ \end{matrix}\right] = \left[\begin{matrix} \varepsilon_{xx} & \gamma_{xy}/2 & \gamma_{xz}/2 \\ \gamma_{yx}/2 & \varepsilon_{yy} & \gamma_{yz}/2 \\ \gamma_{zx}/2 & \gamma_{zy}/2 & \varepsilon_{zz} \\ \end{matrix}\right]\,\!

Read more about this topic:  Infinitesimal Strain Theory

Famous quotes containing the words geometric and/or strain:

    “In mathematics he was greater
    Than Tycho Brahe, or Erra Pater:
    For he, by geometric scale,
    Could take the size of pots of ale;
    Resolve, by sines and tangents straight,
    If bread and butter wanted weight;
    And wisely tell what hour o’ th’ day
    The clock doth strike, by algebra.”
    —Samuel Butler (1612–1680)

    “A man who strains himself on the stage is bound, if he is any good, to strain all the people sitting in the stalls.”
    —Bertolt Brecht (1898–1956)