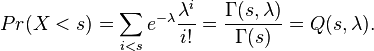Incomplete Gamma Function - Regularized Gamma Functions and Poisson Random Variables

Regularized Gamma Functions and Poisson Random Variables

Two related functions are the regularized Gamma functions:

is the cumulative distribution function for Gamma random variables with shape parameter and scale parameter 1.

When is an integer, is the cumulative distribution function for Poisson random variables: If is a random variable then

Pr(X<s) = \sum_{i<s} e^{-\lambda} \frac{\lambda^i}{i!} = \frac{\Gamma(s,\lambda)}{\Gamma(s)} = Q(s,\lambda).

This formula can be derived by repeated integration by parts.

Read more about this topic:  Incomplete Gamma Function

Famous quotes containing the words functions, random and/or variables:

    “Those things which now most engage the attention of men, as politics and the daily routine, are, it is true, vital functions of human society, but should be unconsciously performed, like the corresponding functions of the physical body.”
    —Henry David Thoreau (1817–1862)

    “We should stop looking to law to provide the final answer.... Law cannot save us from ourselves.... We have to go out and try to accomplish our goals and resolve disagreements by doing what we think is right. That energy and resourcefulness, not millions of legal cubicles, is what was great about America. Let judgment and personal conviction be important again.”
    —Philip K. Howard, U.S. lawyer. The Death of Common Sense: How Law Is Suffocating America, pp. 186-87, Random House (1994)

    “Science is feasible when the variables are few and can be enumerated; when their combinations are distinct and clear. We are tending toward the condition of science and aspiring to do it. The artist works out his own formulas; the interest of science lies in the art of making science.”
    —Paul Valéry (1871–1945)