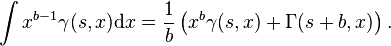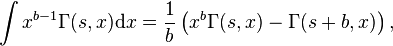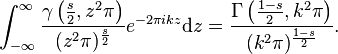Incomplete Gamma Function - Indefinite and Definite Integrals

Indefinite and Definite Integrals

The following indefinite integrals are readily obtained using integration by parts:

\int x^{b-1} \gamma(s,x) \mathrm d x = \frac{1}{b} \left( x^b \gamma(s,x) + \Gamma(s+b,x) \right).
\int x^{b-1} \Gamma(s,x) \mathrm d x = \frac{1}{b} \left( x^b \Gamma(s,x) - \Gamma(s+b,x) \right),

The lower and the upper incomplete Gamma function are connected via the Fourier transform:

\int_{-\infty}^\infty \frac {\gamma\left(\frac s 2, z^2 \pi \right)} {(z^2 \pi)^\frac s 2} e^{-2 \pi i k z} \mathrm d z = \frac {\Gamma\left(\frac {1-s} 2, k^2 \pi \right)} {(k^2 \pi)^\frac {1-s} 2}.

This follows, for example, by suitable specialization of (Gradshteyn & Ryzhik 1980, ยง 7.642).

Read more about this topic:  Incomplete Gamma Function

Famous quotes containing the words indefinite and/or definite:

    “For me chemistry represented an indefinite cloud of future potentialities which enveloped my life to come in black volutes torn by fiery flashes, like those which had hidden Mount Sinai. Like Moses, from that cloud I expected my law, the principle of order in me, around me, and in the world.... I would watch the buds swell in spring, the mica glint in the granite, my own hands, and I would say to myself: “I will understand this, too, I will understand everything.””
    —Primo Levi (1919–1987)

    “The real, then, is that which, sooner or later, information and reasoning would finally result in, and which is therefore independent of the vagaries of me and you. Thus, the very origin of the conception of reality shows that this conception essentially involves the notion of a COMMUNITY, without definite limits, and capable of a definite increase of knowledge.”
    —Charles Sanders Peirce (1839–1914)