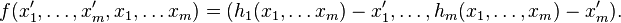Implicit Function Theorem - Application: Change of Coordinates

Application: Change of Coordinates

Suppose we have an m-dimensional space, parametrised by a set of coordinates . We can introduce a new coordinate system by supplying m functions . These functions allow to calculate the new coordinates of a point, given the point's old coordinates using . One might want to verify if the opposite is possible: given coordinates, can we 'go back' and calculate the same point's original coordinates ? The implicit function theorem will provide an answer to this question. The (new and old) coordinates are related by, with

$f(x'_1,\ldots,x'_m,x_1,\ldots x_m)=(h_1(x_1,\ldots x_m)-x'_1,\ldots, h_m(x_1,\ldots, x_m)-x'_m).$

Now the Jacobian matrix of f at a certain point is given by

$\begin{matrix} (Df)(a,b) & = & \begin{bmatrix} -1 & \cdots & 0 & \frac{\partial h_1}{\partial x_1}(b) & \cdots & \frac{\partial h_1}{\partial x_m}(b)\\ \vdots & \ddots & \vdots & \vdots & \ddots & \vdots\\ 0 & \cdots & -1 & \frac{\partial h_m}{\partial x_1}(b) & \cdots & \frac{\partial h_m}{\partial x_m}(b)\\ \end{bmatrix}\\ & = & \begin{bmatrix} -1_m & | & J \end{bmatrix}.\\ \end{matrix}$

where denotes the identity matrix, and J is the matrix of partial derivatives, evaluated at . (In the above, these blocks were denoted by X and Y. As it happens, in this particular application of the theorem, neither matrix depends on .) The implicit function theorem now states that we can locally express as a function of if J is invertible. Demanding J is invertible is equivalent to, thus we see that we can go back from the primed to the unprimed coordinates if the determinant of the Jacobian J is non-zero. This statement is also known as the inverse function theorem.

Read more about this topic: Implicit Function Theorem

Famous quotes containing the word change:

“The will to change begins in the body not in the mind
My politics is in my body, accruing and expanding with every act of resistance and each of my failures.”
—Adrienne Rich (b. 1929)