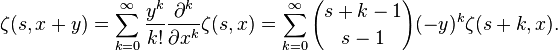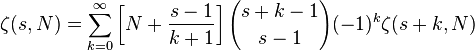Hurwitz Zeta Function - Taylor Series

Taylor Series

The derivative of the zeta in the second argument is a shift:

Thus, the Taylor series has the distinctly umbral form:

\zeta(s,x+y) = \sum_{k=0}^\infty \frac {y^k} {k!} \frac {\partial^k} {\partial x^k} \zeta (s,x) = \sum_{k=0}^\infty {s+k-1 \choose s-1} (-y)^k \zeta (s+k,x).

Closely related is the Stark–Keiper formula:

\zeta(s,N) = \sum_{k=0}^\infty \left {s+k-1 \choose s-1} (-1)^k \zeta (s+k,N)

which holds for integer N and arbitrary s. See also Faulhaber's formula for a similar relation on finite sums of powers of integers.

Read more about this topic:  Hurwitz Zeta Function

Famous quotes containing the words taylor and/or series:

    “Oh, what a might is this whose single frown
    Doth shake the world as it would shake it down?
    Which all from nothing fet, from nothing all;
    Hath all on nothing set, lets nothing fall.
    Gave all to nothing man indeed, whereby
    Through nothing man all might Him glorify.”
    —Edward Taylor (1645–1729)

    “Rosalynn said, “Jimmy, if we could only get Prime Minister Begin and President Sadat up here on this mountain for a few days, I believe they might consider how they could prevent another war between their countries.” That gave me the idea, and a few weeks later, I invited both men to join me for a series of private talks. In September 1978, they both came to Camp David.”
    —Jimmy Carter (James Earl Carter, Jr.)