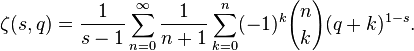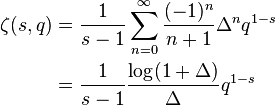Hurwitz Zeta Function - Series Representation

Series Representation

A convergent series representation defined for q > −1 and any complex s ≠ 1 was given by Helmut Hasse in 1930:

$\zeta(s,q)=\frac{1}{s-1} \sum_{n=0}^\infty \frac{1}{n+1} \sum_{k=0}^n (-1)^k {n \choose k} (q+k)^{1-s}.$

This series converges uniformly on compact subsets of the s-plane to an entire function. The inner sum may be understood to be the nth forward difference of ; that is,

where Δ is the forward difference operator. Thus, one may write

$\begin{align} \zeta(s, q) &= \frac{1}{s-1}\sum_{n=0}^\infty \frac{(-1)^n}{n+1} \Delta^n q^{1-s}\\ &= \frac{1}{s-1} {\log(1 + \Delta) \over \Delta} q^{1-s} \end{align}$

Read more about this topic: Hurwitz Zeta Function

Famous quotes containing the word series:

“The woman’s world ... is shown as a series of limited spaces, with the woman struggling to get free of them. The struggle is what the film is about; what is struggled against is the limited space itself. Consequently, to make its point, the film has to deny itself and suggest it was the struggle that was wrong, not the space.”
—Jeanine Basinger (b. 1936)