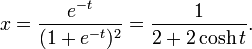Shape
The prototypical Hubbert curve is a probability density function of a logistic distribution curve. It is not a gaussian function (which is used to plot normal distributions), but the two have a similar appearance. The density of a Hubbert curve approaches zero more slowly than a gaussian function:
The graph of a Hubbert curve consists of three key elements:
- a gradual rise from zero resource production that then increases quickly
- a "Hubbert peak", representing the maximum production level
- a drop from the peak that then follows a steep production decline.
The actual shape of a graph of real world production trends is determined by various factors, such as development of enhanced production techniques, availability of competing resources, and government regulations on production or consumption. Because of such factors, real world Hubbert curves are often not symmetrical.
Read more about this topic: Hubbert Curve
Famous quotes containing the word shape:
“Man is not merely the sum of his masks. Behind the shifting face of personality is a hard nugget of self, a genetic gift.... The self is malleable but elastic, snapping back to its original shape like a rubber band. Mental illness is no myth, as some have claimed. It is a disturbance in our sense of possession of a stable inner self that survives its personae.”
—Camille Paglia (b. 1947)
“The structure was designed by an old sea captain who believed that the world would end in a flood. He built a home in the traditional shape of the Ark, inverted, with the roof forming the hull of the proposed vessel. The builder expected that the deluge would cause the house to topple and then reverse itself, floating away on its roof until it should land on some new Ararat.”
—For the State of New Jersey, U.S. public relief program (1935-1943)
“Different persons growing up in the same language are like different bushes trimmed and trained to take the shape of identical elephants. The anatomical details of twigs and branches will fulfill the elephantine form differently from bush to bush, but the overall outward results are alike.”
—Willard Van Orman Quine (b. 1908)