Hohmann Transfer Orbit - Calculation

Calculation

For a small body orbiting another, very much larger body (such as a satellite orbiting the earth), the total energy of the body is the sum of its kinetic energy and potential energy, and this total energy also equals half the potential at the average distance, (the semi-major axis):

Solving this equation for velocity results in the Vis-viva equation,

where:
  • is the speed of an orbiting body
  • is the standard gravitational parameter of the primary body, assuming is not significantly bigger than (which makes )
  • is the distance of the orbiting body from the primary focus
  • is the semi-major axis of the body's orbit.

Therefore the delta-v required for the Hohmann transfer can be computed as follows, under the assumption of instantaneous impulses:

\Delta v_1 = \sqrt{\frac{\mu}{r_1}} \left( \sqrt{\frac{2 r_2}{r_1+r_2}} - 1 \right),
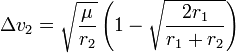\Delta v_2 = \sqrt{\frac{\mu}{r_2}} \left( 1 - \sqrt{\frac{2 r_1}{r_1+r_2}}\,\! \right) ,

where and are, respectively, the radii of the departure and arrival circular orbits; the smaller (greater) of and corresponds to the periapsis distance (apoapsis distance) of the Hohmann elliptical transfer orbit. The total is then:

\Delta v_{total} = \Delta v_1 + \Delta v_2.

Whether moving into a higher or lower orbit, by Kepler's third law, the time taken to transfer between the orbits is:

t_H = \begin{matrix}\frac12\end{matrix} \sqrt{\frac{4\pi^2 a^3_H}{\mu}} = \pi \sqrt{\frac {(r_1 + r_2)^3}{8\mu}}

(one half of the orbital period for the whole ellipse), where is length of semi-major axis of the Hohmann transfer orbit.

Read more about this topic:  Hohmann Transfer Orbit

Famous quotes containing the word calculation:

    “Common sense is the measure of the possible; it is composed of experience and prevision; it is calculation appled to life.”
    —Henri-Frédéric Amiel (1821–1881)

    ““To my thinking” boomed the Professor, begging the question as usual, “the greatest triumph of the human mind was the calculation of Neptune from the observed vagaries of the orbit of Uranus.”
    “And yours,” said the P.B.”
    —Samuel Beckett (1906–1989)