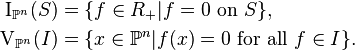Hilbert's Nullstellensatz - Projective Nullstellensatz

We can formulate a certain correspondence between homogeneous ideals of polynomials and algebraic subsets of a projective space, called the projective Nullstellensatz, that is analogous to the affine one. To do that, we introduce some notations. Let The homogeneous ideal is called the maximal homogeneous ideal (see also irrelevant ideal). As in the affine case, we let: for a subset and a homogeneous ideal I of R,

$\begin{align} \operatorname{I}_{\mathbb{P}^n}(S) &= \{ f \in R_+ | f = 0 \text{ on } S \}, \\ \operatorname{V}_{\mathbb{P}^n}(I) &= \{ x \in \mathbb{P}^n | f(x) = 0 \text{ for all }f \in I \}. \end{align}$

By we mean: for every homogeneous coordinates of a point of S we have . This implies that the homogeneous components of f are also zero on S and thus that is a homogeneous ideal. Equivalently, is a homogeneous ideal generated by nonzero homogeneous polynomials f that vanishes on S. Now, for any homogeneous ideal, by the usual Nullstellensatz, we have:

and so, like in the affine case, we have:

There exists an order-reversing one-to-one correspondence between proper homogeneous radical ideals of R and subsets of of the form The correspondence is given by and

Read more about this topic: Hilbert's Nullstellensatz