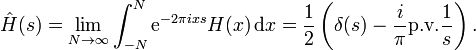Heaviside Step Function - Fourier Transform

Fourier Transform

The Fourier transform of the Heaviside step function is a distribution. Using one choice of constants for the definition of the Fourier transform we have

\hat{H}(s) = \lim_{N\to\infty}\int^N_{-N} \mathrm{e}^{-2\pi i x s} H(x)\,{\rm d}x = \frac{1}{2} \left( \delta(s) - \frac{i}{\pi}\text{p.v.}\frac{1}{s} \right).

Here is the distribution that takes a test function to the Cauchy principal value of The limit appearing in the integral is also taken in the sense of (tempered) distributions.

Read more about this topic:  Heaviside Step Function

Famous quotes containing the word transform:

    “He had said that everything possessed
    The power to transform itself, or else,
    And what meant more, to be transformed.”
    —Wallace Stevens (1879–1955)