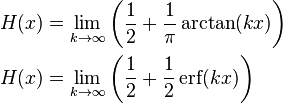Heaviside Step Function - Analytic Approximations

Analytic Approximations

For a smooth approximation to the step function, one can use the logistic function

where a larger k corresponds to a sharper transition at x = 0. If we take H(0) = ½, equality holds in the limit:

There are many other smooth, analytic approximations to the step function. Among the possibilities are:

\begin{align} H(x) &= \lim_{k \rightarrow \infty} \left(\frac{1}{2} + \frac{1}{\pi}\arctan(kx)\right)\\ H(x) &= \lim_{k \rightarrow \infty}\left(\frac{1}{2} + \frac{1}{2}\operatorname{erf}(kx)\right) \end{align}

These limits hold pointwise and in the sense of distributions. In general, however, pointwise convergence need not imply distributional convergence, and vice-versa distributional convergence need not imply pointwise convergence.

In general, any cumulative distribution function (c.d.f.) of a continuous probability distribution that is peaked around zero and has a parameter that controls for variance can serve as an approximation, in the limit as the variance approaches zero. For example, all three of the above approximations are c.d.f.s of common probability distributions: The logistic, Cauchy and normal distributions, respectively.

Read more about this topic:  Heaviside Step Function

Famous quotes containing the word analytic:

    ““You, that have not lived in thought but deed,
    Can have the purity of a natural force,
    But I, whose virtues are the definitions
    Of the analytic mind, can neither close
    The eye of the mind nor keep my tongue from speech.””
    —William Butler Yeats (1865–1939)