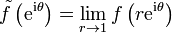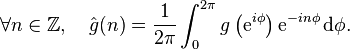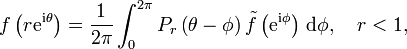Hardy Space - Hardy Spaces On The Unit Circle

Hardy Spaces On The Unit Circle

The Hardy spaces defined in the preceding section can also be viewed as certain closed vector subspaces of the complex Lp spaces on the unit circle. This connection is provided by the following theorem (Katznelson 1976, Thm 3.8): Given f ∈ Hp, with p > 0, the radial limit

\tilde f\left(\mathrm{e}^{\mathrm{i}\theta}\right) = \lim_{r\to 1} f\left(r \mathrm{e}^{\mathrm{i}\theta}\right)

exists for almost every θ. The function belongs to the Lp space for the unit circle, and one has that

Denoting the unit circle by T, and by Hp(T) the vector subspace of Lp(T) consisting of all limit functions, when f varies in Hp, one then has that for p ≥ 1,

(Katznelson 1976), where the ĝ(n) are the Fourier coefficients of a function g integrable on the unit circle,

\forall n \in \mathbb{Z}, \ \ \ \hat{g}(n) = \frac{1}{2\pi}\int_0^{2\pi} g\left(\mathrm{e}^{i\phi}\right) \mathrm{e}^{-in\phi} \, \mathrm{d}\phi.

The space Hp(T) is a closed subspace of Lp(T). Since Lp(T) is a Banach space (for 1 ≤ p ≤ ∞), so is Hp(T).

The above can be turned around. Given a function ∈ Lp(T), with p ≥ 1, one can regain a (harmonic) function f on the unit disk by means of the Poisson kernel Pr:

f\left(r \mathrm{e}^{\mathrm{i}\theta}\right)= \frac{1}{2\pi} \int_0^{2\pi} P_r\left(\theta-\phi\right) \tilde f\left(\mathrm{e}^{\mathrm{i}\phi}\right) \, \mathrm{d}\phi, \ \ \ r < 1,

and f belongs to Hp exactly when is in Hp(T). Supposing that is in Hp(T). i.e. that has Fourier coefficients (an)n ∈ Z with an = 0 for every n < 0, then the element f of the Hardy space Hp associated to is the holomorphic function

In applications, those functions with vanishing negative Fourier coefficients are commonly interpreted as the causal solutions. Thus, the space H2 is seen to sit naturally inside L2 space, and is represented by infinite sequences indexed by N; whereas L2 consists of bi-infinite sequences indexed by Z.

Read more about this topic:  Hardy Space

Famous quotes containing the words hardy, spaces, unit and/or circle:

    “A well-proportioned mind is one which shows no particular bias; one of which we may safely say that it will never cause its owner to be confined as a madman, tortured as a heretic, or crucified as a blasphemer. Also, on the other hand, that it will never cause him to be applauded as a prophet, revered as a priest, or exalted as a king. Its usual blessings are happiness and mediocrity.”
    —Thomas Hardy (1840–1928)

    “In any case, raw aggression is thought to be the peculiar province of men, as nurturing is the peculiar province of women.... The psychologist Erik Erikson discovered that, while little girls playing with blocks generally create pleasant interior spaces and attractive entrances, little boys are inclined to pile up the blocks as high as they can and then watch them fall down: “the contemplation of ruins,” Erikson observes, “is a masculine specialty.””
    —Joyce Carol Oates (b. 1938)

    “During the Suffragette revolt of 1913 I ... [urged] that what was needed was not the vote, but a constitutional amendment enacting that all representative bodies shall consist of women and men in equal numbers, whether elected or nominated or coopted or registered or picked up in the street like a coroner’s jury. In the case of elected bodies the only way of effecting this is by the Coupled Vote. The representative unit must not be a man or a woman but a man and a woman.”
    —George Bernard Shaw (1856–1950)

    “we are the circle of the crazy ladies
    who sit in the lounge of the mental house
    and smile at the smiling woman
    who passes us each a bell,”
    —Anne Sexton (1928–1974)