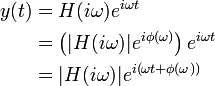Group Delay and Phase Delay - Introduction

Introduction

Group delay is a useful measure of time distortion, and is calculated by differentiating, with respect to frequency, the phase response versus frequency of the device under test (DUT). The group delay is a measure of the slope of the phase response at any given frequency. Variations in group delay cause signal distortion, just as deviations from linear phase cause distortion.

In LTI system theory, control theory, and in digital or analog signal processing, the relationship between the input signal, to output signal, of an LTI system is governed by the convolution operation:

Or, in the frequency domain,

where

and

.

Here is the time-domain impulse response of the LTI system and, are the Laplace transforms of the input, output, and impulse response, respectively. is called the transfer function of the LTI system and, as does the impulse response, fully defines the input-output characteristics of the LTI system.

When such a system is driven by a quasi-sinusoidal signal, (a sinusoid with a slowly changing amplitude envelope, relative to the rate of change of phase, of the sinusoid),

the output of such an LTI system is very well approximated as

if

and and, the group delay and phase delay respectively, are equal to the expressions below (and potentially are functions of angular frequency ω). In a linear phase system (with non-inverting gain), both and are constant and equal to the same overall delay of the system and the unwrapped phase shift of the system is negative with magnitude increasing linearly with frequency ω.

It can be shown that for an LTI system with transfer function driven by a complex sinusoid of unit amplitude,

the output is

\begin{align} y(t) & = H(i \omega) e^{i \omega t} \ \\ & = \left( |H(i \omega)| e^{i \phi(\omega)} \right) e^{i \omega t} \ \\ & = |H(i \omega)| e^{i \left(\omega t + \phi(\omega) \right)} \ \\ \end{align} \

where the phase shift is

Additionally, it can be shown that the group delay, and phase delay, are related to the phase shift as

.

Read more about this topic:  Group Delay And Phase Delay

Famous quotes containing the word introduction:

    “My objection to Liberalism is this—that it is the introduction into the practical business of life of the highest kind—namely, politics—of philosophical ideas instead of political principles.”
    —Benjamin Disraeli (1804–1881)

    “For better or worse, stepparenting is self-conscious parenting. You’re damned if you do, and damned if you don’t.”
    —Anonymous Parent. Making It as a Stepparent, by Claire Berman, introduction (1980, repr. 1986)

    “The role of the stepmother is the most difficult of all, because you can’t ever just be. You’re constantly being tested—by the children, the neighbors, your husband, the relatives, old friends who knew the children’s parents in their first marriage, and by yourself.”
    —Anonymous Stepparent. Making It as a Stepparent, by Claire Berman, introduction (1980, repr. 1986)