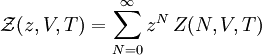Grand Canonical Ensemble - The Partition Function

The Partition Function

Classically, the partition function of the grand canonical ensemble is given as a weighted sum of canonical partition functions with different number of particles ,

$\mathcal{Z}(z, V, T) = \sum_{N=0}^{\infty} z^N \, Z(N, V, T) \,$

where is defined below, and denotes the partition function of the canonical ensemble at temperature, of volume, and with the number of particles fixed at . (In the last step, we have expanded the canonical partition function, and is the Boltzmann constant, the second sum is performed over all microscopic states, denoted by with energy . )

Quantum mechanically, the situation is even simpler (conceptually). For a system of bosons or fermions, it is often mathematically easier to treat the number of particles of the system as an intrinsic property of each quantum (eigen-)state, . Therefore the partition function can be written as

The parameter is called fugacity, and it represents the ease of adding a new particle into the system. The chemical potential (or Gibbs free energy per particle) is directly related to the fugacity through

Note that both fugacity and the chemical potential control the number of particles in a system (just as temperature controls the energy). However, we have used fugacity, rather than chemical potential, in defining the partition function because fugacity is independent of temperature, while the chemical potential contains temperature dependence.

Read more about this topic: Grand Canonical Ensemble

Famous quotes containing the word function:

“For me being a poet is a job rather than an activity. I feel I have a function in society, neither more nor less meaningful than any other simple job. I feel it is part of my work to make poetry more accessible to people who have had their rights withdrawn from them.”
—Jeni Couzyn (b. 1942)