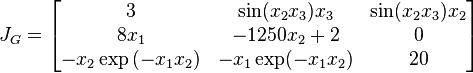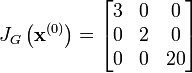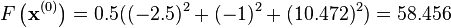Gradient Descent - Solution of A Non-linear System

Solution of A Non-linear System

Gradient descent can also be used to solve a system of nonlinear equations. Below is an example that shows how to use the gradient descent to solve for three unknown variables, x1, x2, and x3. This example shows one iteration of the gradient descent.

Consider a nonlinear system of equations:

\begin{cases} 3x_1-\cos(x_2x_3)-\tfrac{3}{2}=0 \\ 4x_1^2-625x_2^2+2x_2-1=0 \\ \exp(-x_1x_2)+20x_3+\tfrac{10\pi-3}{3}=0 \end{cases}

suppose we have the function

G(\mathbf{x}) = \begin{bmatrix} 3x_1-\cos(x_2x_3)-\tfrac{3}{2} \\ 4x_1^2-625x_2^2+2x_2-1 \\ \exp(-x_1x_2)+20x_3+\tfrac{10\pi-3}{3} \\ \end{bmatrix}

where

\mathbf{x} =\begin{bmatrix} x_1 \\ x_2 \\ x_3 \\ \end{bmatrix}

and the objective function

With initial guess

\mathbf{x}^{(0)}=\begin{bmatrix} x_1 \\ x_2 \\ x_3 \\ \end{bmatrix} =\begin{bmatrix} 0 \\ 0 \\ 0 \\ \end{bmatrix}

We know that

where

The Jacobian matrix

J_G = \begin{bmatrix} 3 & \sin(x_2x_3)x_3 & \sin(x_2x_3)x_2 \\ 8x_1 & -1250x_2+2 & 0 \\ -x_2\exp{(-x_1x_2)} & -x_1\exp(-x_1x_2) & 20\\ \end{bmatrix}

Then evaluating these terms at

J_G \left(\mathbf{x}^{(0)}\right) = \begin{bmatrix} 3 & 0 & 0\\ 0 & 2 & 0\\ 0 & 0 & 20 \end{bmatrix}

and

G(\mathbf{x}^{(0)}) = \begin{bmatrix} -2.5\\ -1\\ 10.472 \end{bmatrix}

So that

\mathbf{x}^{(1)}=0-\gamma_0 \begin{bmatrix} -7.5\\ -2\\ 209.44 \end{bmatrix}.

and

F \left(\mathbf{x}^{(0)}\right) = 0.5((-2.5)^2 + (-1)^2 + (10.472)^2) = 58.456

Now a suitable must be found such that . This can be done with any of a variety of line search algorithms. One might also simply guess which gives

\mathbf{x}^{(1)}=\begin{bmatrix} 0.0075 \\ 0.002 \\ -0.20944 \\ \end{bmatrix}

evaluating at this value,

F \left(\mathbf{x}^{(1)}\right) = 0.5((-2.48)^2 + (-1.00)^2 + (6.28)^2) = 23.306

The decrease from to the next step's value of is a sizable decrease in the objective function. Further steps would reduce its value until a solution to the system was found.

Read more about this topic:  Gradient Descent

Famous quotes containing the words solution of, solution and/or system:

    “Give a scientist a problem and he will probably provide a solution; historians and sociologists, by contrast, can offer only opinions. Ask a dozen chemists the composition of an organic compound such as methane, and within a short time all twelve will have come up with the same solution of CH4. Ask, however, a dozen economists or sociologists to provide policies to reduce unemployment or the level of crime and twelve widely differing opinions are likely to be offered.”
    —Derek Gjertsen, British scientist, author. Science and Philosophy: Past and Present, ch. 3, Penguin (1989)

    “There’s one solution that ends all life’s problems.”
    —Chinese proverb.

    “Books are for the most part willfully and hastily written, as parts of a system to supply a want real or imagined.”
    —Henry David Thoreau (1817–1862)