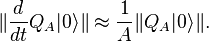Goldstone Boson - Goldstone's Argument

Goldstone's Argument

The principle behind Goldstone's argument is that the ground state is not unique. Normally, by current conservation, the charge operator for any symmetry current is time-independent,

{d\over dt} Q = {d\over dt} \int_x J^0(x) =0 ~.

Acting with the charge operator on the vacuum either annihilates the vacuum, if that is symmetric; else, if not, as is the case in spontaneous symmetry breaking, it produces a zero-frequency state out of it, through its shift transformation feature illustrated above. (Actually, here, the charge itself is ill-defined; but its better behaved commutators with fields, so, then, the transformation shifts, are still time-invariant, d〈δφg〉/dt = 0 .)

So, if the vacuum is not invariant under the symmetry, action of the charge operator produces a state which is different from the vacuum chosen, but which has zero frequency. This is a long-wavelength oscillation of a field which is nearly stationary: there are states with zero frequency, so that the theory cannot have a mass gap.

This argument is clarified by taking the limit carefully. If an approximate charge operator is applied to the vacuum,

{d\over dt} Q_A = {d\over dt} \int_x e^{-x^2\over 2A^2} J^0(x) = -\int_x e^{-x^2\over 2A^2} \nabla \cdot J = \int_x \nabla(e^{-x^2\over 2A^2}) \cdot J ~ ,

a state with approximately vanishing time derivative is produced,

\| {d\over dt} Q_A |0\rangle \| \approx {1\over A} \| Q_A|0\rangle \|.

Assuming a nonvanishing mass gap m0, the frequency of any state like the above, which is orthogonal to the vacuum, is at least m0,

\| {d\over dt} |\theta\rangle \| = \| H |\theta\rangle \| \ge m_0 \| \; |\theta\rangle \| ~.

Letting A become large leads to a contradiction. Consequently m0 = 0.

This argument fails, however, when the symmetry is gauged, because then the symmetry generator is only performing a gauge transformation. A gauge transformed state is the same exact state, so that acting with a symmetry generator does not get one out of the vacuum.

Read more about this topic:  Goldstone Boson

Famous quotes containing the word argument:

    “Our argument ... will result, not upon logic by itself—though without logic we should never have got to this point—but upon the fortunate contingent fact that people who would take this logically possible view, after they had really imagined themselves in the other man’s position, are extremely rare.”
    —Richard M. Hare (b. 1919)