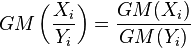Properties
The fundamental property of the geometric mean, which can be proven to be false for any other mean, is
This makes the geometric mean the only correct mean when averaging normalized results, that is results that are presented as ratios to reference values. This is the case when presenting computer performance with respect to a reference computer, or when computing a single average index from several heterogeneous sources (for example life expectancy, education years and infant mortality). In this scenario, using the arithmetic or harmonic mean would change the ranking of the results depending on what is used as a reference. For example, take the following comparison of execution time of computer programs:
| Computer A | Computer B | Computer C | |
|---|---|---|---|
| Program 1 | 1 | 10 | 20 |
| Program 2 | 1000 | 100 | 20 |
| Arithmetic mean | 500.5 | 55 | 20 |
| Geometric mean | 31.622 . . . | 31.622 . . . | 20 |
The arithmetic and geometric means "agree" that computer C is the fastest. However, by presenting appropriately normalized values and using the arithmetic mean, we can show either of the other two computers to be the fastest. Normalizing by A's result gives A as the fastest computer according to the arithmetic mean:
| Computer A | Computer B | Computer C | |
|---|---|---|---|
| Program 1 | 1 | 10 | 20 |
| Program 2 | 1 | 0.1 | 0.02 |
| Arithmetic mean | 1 | 5.05 | 10.01 |
| Geometric mean | 1 | 1 | 0.632 . . . |
while normalizing by B's result gives B as the fastest computer according to the arithmetic mean:
| Computer A | Computer B | Computer C | |
|---|---|---|---|
| Program 1 | 0.1 | 1 | 2 |
| Program 2 | 10 | 1 | 0.2 |
| Arithmetic mean | 5.05 | 1 | 1.1 |
| Geometric mean | 1 | 1 | 0.632 |
In all cases, the ranking given by the geometric mean stays the same as the one obtained with unnormalized values.
Read more about this topic: Geometric Mean
Famous quotes containing the word properties:
“The reason why men enter into society, is the preservation of their property; and the end why they choose and authorize a legislative, is, that there may be laws made, and rules set, as guards and fences to the properties of all the members of the society: to limit the power, and moderate the dominion, of every part and member of the society.”
—John Locke (1632–1704)
“A drop of water has the properties of the sea, but cannot exhibit a storm. There is beauty of a concert, as well as of a flute; strength of a host, as well as of a hero.”
—Ralph Waldo Emerson (1803–1882)