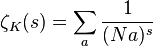Generalized Riemann Hypothesis - Extended Riemann Hypothesis (ERH)

Extended Riemann Hypothesis (ERH)

Suppose K is a number field (a finite-dimensional field extension of the rationals Q) with ring of integers OK (this ring is the integral closure of the integers Z in K). If a is an ideal of OK, other than the zero ideal we denote its norm by Na. The Dedekind zeta-function of K is then defined by

\zeta_K(s) = \sum_a \frac{1}{(Na)^s}

for every complex number s with real part > 1. The sum extends over all non-zero ideals a of OK.

The Dedekind zeta-function satisfies a functional equation and can be extended by analytic continuation to the whole complex plane. The resulting function encodes important information about the number field K. The extended Riemann hypothesis asserts that for every number field K and every complex number s with ΞΆK(s) = 0: if the real part of s is between 0 and 1, then it is in fact 1/2.

The ordinary Riemann hypothesis follows from the extended one if one takes the number field to be Q, with ring of integers Z.

Read more about this topic:  Generalized Riemann Hypothesis

Famous quotes containing the words extended and/or hypothesis:

    “No: until I want the protection of Massachusetts to be extended to me in some distant Southern port, where my liberty is endangered, or until I am bent solely on building up an estate at home by peaceful enterprise, I can afford to refuse allegiance to Massachusetts, and her right to my property and life. It costs me less in every sense to incur the penalty of disobedience to the State than it would to obey. I should feel as if I were worth less in that case.”
    —Henry David Thoreau (1817–1862)

    “Oversimplified, Mercier’s Hypothesis would run like this: “Wit is always absurd and true, humor absurd and untrue.””
    —Vivian Mercier (b. 1919)