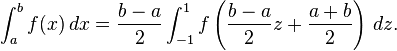Gaussian Quadrature - Change of Interval

Change of Interval

An integral over must be changed into an integral over before applying the Gaussian quadrature rule. This change of interval can be done in the following way:

\int_a^b f(x)\,dx = \frac{b-a}{2} \int_{-1}^1 f\left(\frac{b-a}{2}z + \frac{a+b}{2}\right)\,dz.

After applying the Gaussian quadrature rule, the following approximation is:

\int_a^b f(x)\,dx \approx \frac{b-a}{2} \sum_{i=1}^n w_i f\left(\frac{b-a}{2}z_i + \frac{a+b}{2}\right).

Read more about this topic:  Gaussian Quadrature

Famous quotes containing the words change and/or interval:

    “... often in the heat of noonday, leaning on a hoe, looking across valleys at the mountains, so blue, so close, my only conscious thought was, “How can I ever get away from here? How can I get to where they have books, where I can be educated?” I worked hard, always waiting for something to happen to change things. There came a time when I knew I must make them happen; that no one would do anything about it for me. And I did.”
    —Belinda Jelliffe (1892–1979)

    “[I have] been in love with one princess or another almost all my life, and I hope I shall go on so, till I die, being firmly persuaded, that if ever I do a mean action, it must be in some interval betwixt one passion and another.”
    —Laurence Sterne (1713–1768)