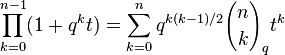Gaussian Binomial Coefficient - Properties

Properties

Like the ordinary binomial coefficients, the Gaussian binomial coefficients are center-symmetric i.e. invariant under the reflection :

In particular,

The name Gaussian binomial coefficient stems from the fact that their evaluation at q = 1 is

for all m and r.

The analogs of Pascal identities for the Gaussian binomial coefficients are

and

There are analogs of the binomial formula, and of Newton's generalized version of it for negative integer exponents, although for the former the Gaussian binomial coefficients themselves do not appear as coefficients:

\prod_{k=0}^{n-1} (1+q^kt)=\sum_{k=0}^n q^{k(k-1)/2} {n \choose k}_q t^k

and

\prod_{k=0}^{n-1} \frac{1}{(1-q^kt)}=\sum_{k=0}^\infty {n+k-1 \choose k}_q t^k.

which, for become:

and

\prod_{k=0}^\infty \frac{1}{(1-q^kt)}=\sum_{k=0}^\infty \frac{t^k}{_q!\,(1-q)^k} .

The first Pascal identity allows one to compute the Gaussian binomial coefficients recursively (with respect to m ) using the initial "boundary" values

and also incidentally shows that the Gaussian binomial coefficients are indeed polynomials (in q). The second Pascal identity follows from the first using the substitution and the invariance of the Gaussian binomial coefficients under the reflection . Both Pascal identities together imply

which leads (when applied iteratively for m, m − 1, m − 2,....) to an expression for the Gaussian binomial coefficient as given in the definition above.

Read more about this topic:  Gaussian Binomial Coefficient

Famous quotes containing the word properties:

    “A drop of water has the properties of the sea, but cannot exhibit a storm. There is beauty of a concert, as well as of a flute; strength of a host, as well as of a hero.”
    —Ralph Waldo Emerson (1803–1882)

    “The reason why men enter into society, is the preservation of their property; and the end why they choose and authorize a legislative, is, that there may be laws made, and rules set, as guards and fences to the properties of all the members of the society: to limit the power, and moderate the dominion, of every part and member of the society.”
    —John Locke (1632–1704)