Fractional Calculus - Heuristics

Heuristics

A fairly natural question to ask is whether there exists an operator, or half-derivative, such that

.

It turns out that there is such an operator, and indeed for any, there exists an operator such that

,

or to put it another way, the definition of can be extended to all real values of n.

Assuming a function that is defined where, form the definite integral from 0 to x. Call this

.

Repeating this process gives

,

and this can be extended arbitrarily.

The Cauchy formula for repeated integration, namely

leads in a straightforward way to a generalization for real n.

Using the Gamma function to remove the discrete nature of the factorial function gives us a natural candidate for fractional applications of the integral operator.

This is in fact a well-defined operator.

It is straightforward to show that the J operator satisfies

Proof

\begin{align} (J^\alpha) (J^\beta f)(x) & = \frac{1}{\Gamma(\alpha)} \int_0^x (x-t)^{\alpha-1} (J^\beta f)(t) \; dt \\ & = \frac{1}{\Gamma(\alpha) \Gamma(\beta)} \int_0^x \int_0^t (x-t)^{\alpha-1} (t-s)^{\beta-1} f(s) \; ds \; dt \\ & = \frac{1}{\Gamma(\alpha) \Gamma(\beta)} \int_0^x f(s) \left( \int_s^x (x-t)^{\alpha-1} (t-s)^{\beta-1} \; dt \right) ds \end{align}

where in the last step we exchanged the order of integration and pulled out the f(s) factor from the t integration. Changing variables to r defined by t=s+(x-s)r,

(J^\alpha) (J^\beta f)(x) = \frac{1}{\Gamma(\alpha) \Gamma(\beta)} \int_0^x (x-s)^{\alpha + \beta - 1} f(s) \left( \int_0^1 (1-r)^{\alpha-1} r^{\beta-1} \; dr \right) ds

The inner integral is the beta function which satisfies the following property

\int_0^1 (1-r)^{\alpha-1} r^{\beta-1} \; dr = B(\alpha, \beta) = \dfrac{\Gamma(\alpha)\,\Gamma(\beta)}{\Gamma(\alpha+\beta)}

Substituting back into our equation

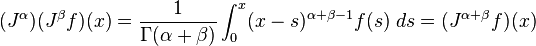
(J^\alpha) (J^\beta f)(x) = \frac{1}{\Gamma(\alpha + \beta)} \int_0^x (x-s)^{\alpha + \beta - 1} f(s) \; ds = (J^{\alpha + \beta} f)(x)

Interchanging α and β shows that the order in which the J operator is applied is irrelevant and completes the proof.

This relationship is called the semigroup property of fractional differintegral operators. Unfortunately the comparable process for the derivative operator D is significantly more complex, but it can be shown that D is neither commutative nor additive in general.

Read more about this topic:  Fractional Calculus